i_NRACM: incorporating ¹⁵N into the Regional Atmospheric Chemistry Mechanism (RACM) for assessing the role photochemistry plays in controlling the isotopic composition of NO_x, NO_y, and atmospheric nitrate

Nitrogen oxides, classified as NO_x (nitric oxide (NO) + nitrogen dioxide (NO₂)) and NO_y (NO_x+ NO₃, N₂O₅ HNO₃, + HNO₄+ HONO + Peroxyacetyl nitrate (PAN) + organic nitrates + any oxidized N compound), are important trace gases in the troposphere, which play an important role in the formation of ozone, particulate matter (PM), and secondary organic aerosols (SOA). There remain many uncertainties in the origin and fate of atmospheric N compounds including the understanding of NO_y cycling, NO_x emission budgets, unresolved issues within the heterogeneous uptake coefficients of N₂O₅, and the formation of organic nitrates in urban forests, to name a few. A potential tool to resolve some of these uncertainties are using natural abundance N isotopes in NO_y compounds. Here we have developed a photochemical mechanism used to simulate tropospheric photochemistry to include ¹⁵N compounds and reactions as a means to simulate δ¹⁵N values in NO_y compounds. The 16 N compounds and 96 reactions involving N used in the Regional Atmospheric Chemistry Mechanism (RACM) were replicated using ¹⁵N in a new mechanism called i_NRACM. The 192 N reactions in i_NRACM were tested to see if isotope effects were relevant with respect to significantly changing the δ¹⁵N values (±1 ‰) of NO_x, HONO, and/or HNO₃. The isotope fractionation factors (α) for relevant reactions were assigned based on recent experimental or calculated values. Each relevant reaction in the i_NRACM mechanism was tested individually and in concert in order to assess the controlling reactions. The controlling reactions and their diurnal importance are discussed. A comparison between i_NRACM predictions and observed δ¹⁵N NO${}_{\mathrm{3}}^{-}$ in particulate matter from Tucson, Arizona, suggests the model, and isotope fractionation factors incorporated into it, are accurately capturing the isotope effects occurring during the photochemistry of NO_y. The implication is that measurements of δ¹⁵N in NO_y compounds may be a new way of tracing in situ N chemistry and a means of assessing NO_x emission budgets.

1 Introduction

Nitrogen oxides are an integral part of atmospheric chemistry, controlling the oxidation state of the troposphere, influencing aerosol formation, altering the pH of rainwater, and facilitating the movement of nitrogen through the N cycle. Nitrogen oxides are classified as NO_x (nitric oxide (NO) + nitrogen dioxide (NO₂)) and NO_y (NO_x+ NO₃, N₂O₅ HNO₃, + HNO₄+ HONO + Peroxyacetyl nitrate (PAN) + organic nitrates + any oxidized N compound) (Day et al., 2003; Hegglin et al., 2006; Ma et al., 2013). NO_x produces ozone (O₃) through NO₂ photolysis, and NO_x acts as a catalyst in O₃ production when volatile organic compounds (VOCs) are present. In turn, O₃ photolysis generates OH radicals, which initiates a radical chain reaction involving HO₂ and organic peroxide propagators that results in the oxidation of chemically reduced compounds in the troposphere making them more soluble (Finlayson-Pitts and Pitts, 2000; Seinfeld and Pandis, 1998). Thus, NO_x facilitates the cleansing of the atmosphere through the production of O₃ and OH_x (OH + HO₂), which together define the troposphere's oxidation state (Bloss et al., 2005; Lelieveld et al., 2008; Prinn, 2003). These oxidants play an important role in the formation of particulate matter (PM) (Bauer et al., 2007; Pye et al., 2010), forming secondary organic aerosols (SOA) via VOC oxidation (Hoyle et al., 2011; Shrivastava et al., 2017). They also generate secondary inorganic PM through NO_x, sulfur oxide (SO_x), and ammonia (NH₃) neutralization, which leads to ammonium nitrate (NH₄NO₃) and ammonium sulfate ((NH₄)₂SO₄) production (Cao et al., 2017; Pan et al., 2018; Pusede et al., 2016). The production of PM has important consequences for air quality aerosols (Andreae and Crutzen, 1997), human health (Bruningfann and Kaneene, 1993; Hall et al., 1992), and radiative forcing (Charlson et al., 1992; Chen et al., 2007). Termination reactions in NO_y cycling produces HNO₃ and facilitates the production of sulfuric acid (H₂SO₄), two strong acids that decrease the pH of rain, known colloquially as acid rain, and impact aerosol pH, both of which trigger a number of negative impacts on the environment (Brimblecombe et al., 2007; Lajtha and Jones, 2013). When NO_y is deposited to the surface by wet and dry deposition, it transfers bioavailable N to ecosystems that may be bereft of, or saturated with, bioavailable N. This process can shift the balance of both terrestrial and aquatic ecosystems and impact the goods and services that those ecosystems can normally deliver (Du et al., 2019; Elliott et al., 2019; Fowler et al., 2013). Thus, understanding NO_y sources and their chemistry is important for an array of scientific disciplines and evaluating their social, economic, and cultural impact on the environment.

Despite this importance, there are numerous knowledge gaps in the understanding of the cycling of NO_y in the atmosphere. The NO_x emission budget is still poorly constrained. Most emission inventories rely on fixed emission factors for some sources that may, in fact, be variable. For example, power plant NO_x emissions are based on an assumed efficiency of catalytic converters that may not be accurate (Srivastava et al., 2005; Felix et al., 2012). Soil NO emissions are highly dependent on soils moisture, redox conditions, fertilizer application rates, type, and timing, making them challenging to constrain (Shepherd et al., 1991; Galloway et al., 2004; Hudman et al., 2012; Houlton et al., 2013; Pilegaard, 2013). There are several unresolved issues with the heterogeneous uptake coefficients of N₂O₅ (Brown et al., 2001, 2006; Chang et al., 2011) and the formation of organic nitrates in urban forests (Lee et al., 2016; Romer et al., 2016; Kastler and Ballschmiter, 1998). The relative importance and mechanism of HONO formation versus HONO emissions are also hotly debated. Likewise, reactions of NO_y in the aqueous-phase and mixed aerosols are not fully understood. Chemical transport models (CTMs) do not accurately predict aerosol nitrate concentrations or other NO_y mixing ratios (Spak and Holloway, 2009; Zhang et al., 2009). Therefore, it is important that these uncertainties in NO_y cycling be resolved if we aim to have accurate air quality forecasting and accurate chemistry–climate models that use CTMs.

It has been suggested that stable N isotopes can provide clues as to the origin of NO_x (Elliott et al., 2009; Felix and Elliott, 2014; Walters et al., 2015b) and the oxidation pathways that transform in NO_y (Freyer et al., 1993; Walters and Michalski, 2015, 2016). Isotopic measurements of NO_y compounds show a wide range of δ¹⁵N values (Eq. 1), which has been suggested to indicate variability in NO_x emission sources, chemical processing, and/or a combination of these effects. δ¹⁵N is defined by the relative difference between the ${}^{\mathrm{15}}\mathrm{N}{/}^{\mathrm{14}}\mathrm{N}$ ratio in a NO_y compound and the ratio in air N₂ (the arbitrary reference compound) and is typically reported in parts per thousand, i.e., per mill (‰).

$$\begin{array}{}\text{(1)}& \begin{array}{rl}{\mathit{\delta }}^{\mathrm{15}}{\text{N}}_{{\mathrm{NO}}_y}\left(\mathrm{‰}\right)& =\left[\right({}^{\mathrm{15}}{\mathrm{NO}}_y{/}^{\mathrm{14}}{\mathrm{NO}}_y)/({}^{\mathrm{15}}{\mathrm{N}}_{\mathrm{2}}{/}^{\mathrm{14}}{\mathrm{N}}_{\mathrm{2}})-\mathrm{1}]\\ & \cdot \mathrm{1000}\end{array}\end{array}$$

A number of studies have measured the δ¹⁵N values of NO_x collected from NO_x sources such as power plants (Felix et al., 2012), automobiles (Walters et al., 2015a), biomass burning (Fibiger and Hastings, 2016), and non-road sources (Felix and Elliott, 2014).

Many studies have measured the δ¹⁵N values of various NO_y compounds collected from the troposphere. Most of the NO_y δ¹⁵N data are for nitrate that has been collected on filters (PM_2.5, PM₁₀, TSP) (Moore, 1977; Savard et al., 2017), as the dissolved NO${}_{\mathrm{3}}^{-}$ anion in rain (Heaton, 1987; Hastings et al., 2003; Felix et al., 2015; Yu and Elliott, 2017), or as gas-phase HNO₃ (Elliott et al., 2009; Savard et al., 2017). The range of tropospheric NO_y δ¹⁵N values span −50 ‰ to +15 ‰ but the average is ∼0 ‰. Two hypotheses have been offered to explain these ranges: source and photochemistry. The source hypothesis (Elliott et al., 2007; Hastings et al., 2013) suggests that the tropospheric NO_y δ¹⁵N value range reflects the spatial and temporal mixing of NO_x sources with different δ¹⁵N values that is then converted into NO_y. The photochemistry hypothesis (Freyer, 1991; Freyer et al., 1993; Walters et al., 2018) suggests that the observed NO_y δ¹⁵N variations arise via isotope effects occurring when photochemical cycling partitions N into the myriad of NO_y compounds. These two hypotheses are not mutually exclusive. Indeed, it is likely to be a combination of both processes, but their relative importance likely shifts depending on environmental conditions such as a region's NO_x source diversity, plume versus dispersed chemistry, photolysis intensity, and oxidant load. In turn, the δ¹⁵N data might be a new key to reconciling some of the current uncertainties in NO_y sources and chemistry, if they can be properly interpreted.

What has become clear is that the temporal–spatial heterogeneity of NO_x sources and the complex photochemistry of NO_y presents a serious challenge to deciphering the observed NO_y δ¹⁵N values. Except for a few isolated cases, a proper assessment of NO_y δ¹⁵N values will require incorporating isotope effects into 3-D chemical transport models. This will include emission modeling of ¹⁵NO_x, meteorological mixing, factoring in isotope effects during NO_y removal processes, and developing chemical mechanisms that incorporate ¹⁵N compounds and their relative rate constants. Here we take the first step in this endeavor by developing a chemical mechanism (0-D photochemical box model) that explicitly includes ¹⁵NO_y compounds and the isotope effects that occur during their cycling through photolysis, equilibrium, and kinetic reactions.

2 Methods

2.1 Chemical and isotopic compounds and reactions included in i_NRACM

The i_NRACM model incorporates ¹⁵N into the Regional Atmospheric Chemistry Mechanism (RACM) detailed in Stockwell et al. (1997). RACM is an extension of the chemical mechanism used in the Regional Acid Deposition Model (RADM2) (Stockwell et al., 1990), but with updated inorganic and organic chemistry. There are 17 stable inorganic compounds, 4 inorganic intermediates, 32 stable organic compounds (including 4 biogenic organics), and 24 organic intermediates in the RACM mechanism. The RACM mechanism uses 237 chemical reactions, including 23 photolysis reactions (Atkinson, 1990; Atkinson et al., 1992). The rate constants, photolysis cross sections, and quantum yields for the inorganic compounds were taken from DeMore et al. (1994). The RACM mechanism aggregates the thousands of VOCs in the atmosphere into 16 anthropogenic and 3 biogenic organic compounds. Part of the aggregation criteria is based on the reactivity of a VOC towards the hydroxyl radical (•OH). Full details on how •OH reacts with alkanes, alkenes, aromatics, and other VOCs, and the aggregation rationale, can be found in Stockwell et al. (1997). The actinic flux model used in RACM was developed by Madronich (1987) and calculates the wavelength-dependent photon flux as a function of solar zenith angle, which is a function of time (hourly), date, latitude, and longitude. Inputs to the model include temperature, water vapor content, pressure, initial gas mixing ratios and primary pollutant emission rates. Complete details on the RACM mechanism can be found in Stockwell et al. (1997).

Our i_NRACM (isotope N in RACM) mechanism was generated by adding ¹⁵N isotopologues for the 2 primary (NO, NO₂) and the 14 secondary N pollutants found in the original RACM mechanism (Table S1a). By definition, an isotopologue is a compound with the same chemical formula but a different mass (e.g., NO = 30 amu and ¹⁵NO = 31 amu, with N = ¹⁴N). This is different from isotopomers, which are isotopic isomers, compounds with the same mass but a different structure caused by isotopic substitution (e.g., ¹⁵NNO₅=109 amu and N¹⁵NO₅=109 amu). Of all the reactive N compounds only N₂O₅ has multiple possible ¹⁵N substitutions, and 2 isotopologues were defined in the i_NRACM: ¹⁵NNO₅ and N¹⁵NO₅. The ¹⁵N compounds are numbered (Table S1a) and subscripted (a, b) in order to maintain a compound numbering scheme that is consistent with that in Stockwell et al. (1997). The non-N compounds found in both RACM and i_NRACM mechanisms have been excluded from Table S1a for the sake of brevity but can be found in Stockwell et al. (1997). The 16 ¹⁵N compounds (Table S1a) were added to the original RACM FORTRAN code provided by Stockwell by using Z=¹⁵N (e.g., ¹⁵NO is defined as ZO).

The 96 chemical reactions involving N compounds (Table S2a–f) were inspected and replicated for ¹⁵N based on classification as the reaction being either “N-only” or “multiple-N” reactions. Single N reactions are those where only one N compound was found in the products and reactants, for example NO + O₃ → NO₂ + O₂. Multiple N reactions could have multiple N compounds in the reactants, the products, or both. Examples of these possible multiple N reactions are NO₂ + NO₃ → N₂O₅, N₂O₅ → NO₂ + NO₃, and NO₃ + NO → NO₂ + NO₂ respectively. For these multiple N reactions, a reaction probability was factored into the isotopologue/isotopomer rate constants (discussed below). For example, the N isotopologue/isotopomer equivalent of the N₂O₅ → NO₂ + NO₃ reaction has two isotopomer reactions: ¹⁵NNO₅ →¹⁵NO₂ + NO₃ and ¹⁵NNO₅ → NO₂ + ¹⁵NO₃. These two isotopologue rate constants (R54a, R54b) are multiplied by a factor of $\mathrm{1}/\mathrm{2}$ to account for this statistical probability. Similar statistical factors were considered when N compounds or intermediates decomposed or reacted to form multiple N products (R52a, R52b, R52c, R52d). All N isotopologue reaction stoichiometry is given in Table S2a–f.

2.2 Isotope effects included in i_NRACM

The main challenge for developing realistic isotopologue chemistry in i_NRACM is quantifying the differences in rate constants caused by isotopic substitution. These isotope effects can be classified into four general types: Equilibrium isotope effects (EIEs), kinetic isotope effects (KIEs), photo-induced isotope fractionation effects (PHIFEs), and vapor pressure isotope effects (VPIEs). For this study, the most up-to-date isotope fractionations were used when establishing the framework for modeling their effect associated with NO_x oxidation chemistry. The established framework will easily enable an adjustment of isotope effects as we improve our understanding of isotope fractionation factors.

Urey (1947) and Bigelesien and Mayer (1947) showed that EIEs are driven by the sensitivity of molecular and condensed-phase vibrational frequencies to isotopic substitutions (Bigeleisen and Mayer, 1947; Urey, 1947). Because vibrations are used in the molecular partition function (Q) to calculate equilibrium constants, isotopic substitution results in isotopologues having different equilibrium constants. Urey (1947) defined the reduced partition function ratio for two isotopologues of the same compound as a β value. For example, the reduced partition function ratio of nitric oxide N isotopologues is $Q_{{}^{\mathrm{15}}\mathrm{NO}}/Q_{\mathrm{NO}}={\mathit{\beta }}_{\mathrm{NO}}$, with the heavy isotope placed in the numerator by convention. The ratio of two β values is denoted as ${\mathit{\alpha }}_{\mathit{\beta }\mathrm{1}/\mathit{\beta }\mathrm{2}}$, the isotope fractionation factor. For example, ${\mathit{\alpha }}_{\mathrm{NO}/{\mathrm{NO}}_{\mathrm{2}}}$ is the temperature-dependent isotope fractionation factor (EIE) for NO + ¹⁵NO₂ $\leftarrow \to$ ¹⁵NO + NO₂. In this case, at 298 K β_NO=1.0669, ${\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{2}}}=\mathrm{1.1064}$, and ${\mathit{\alpha }}_{\mathrm{NO}/{\mathrm{NO}}_{\mathrm{2}}}={\mathit{\beta }}_{\mathrm{NO}}/{\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{2}}}=\mathrm{0.9643}$ (Walters and Michalski, 2015).

A KIE is the relative change in the rate of a unidirectional chemical reaction when one of the atoms of the reactants is substituted with an isotope (Bigeleisen and Wolfsberg, 1958). KIEs are driven by the change in energy required to proceed over the reaction barrier (transition state) as well as changes in the probability of quantum mechanical tunneling (Wolfsberg et al., 2010). This generally results in a lighter isotopologue reacting faster than a heavier isotopologue. Much of the early research on KIEs were investigations of the KIE in reactions containing hydrogen isotopes and these studies usually defined a KIE = $k_{\mathrm{L}}/k_{\mathrm{H}}={\mathit{\alpha }}_{\mathrm{L}/\mathrm{H}}$, where the k values are the rate constants for the light and heavy isotopologues. This is the inverse of the definition of α usually used in research dealing with EIE, VPIE, and PHIFE, and this inversion can lead to confusion. In this paper, in order to maintain consistency between the α values for EIE, KIE, VPIE, and PHIFE, α will be defined as heavy or light for all four effects.

The α values for EIE and KIE can be determined using a number of approaches. The α values for EIE can be calculated if molecular constants (e.g., harmonic frequencies and anharmonicity constants) of the isotopologue pair are known. Accurate molecular constants are difficult to accurately measure for large molecules, and as a result, they primarily exist only for diatomic and triatomic isotopologues (Richet et al., 1977). The only experimental EIE values for ¹⁵N isotopologues of NO_y is for the EIE between NO and NO₂ (Sharma et al., 1970; Walters et al., 2016). To determine the EIE in other NO_y compounds we must rely on quantum chemistry computation methods to estimate the molecular constants and anharmonicity constants. Recent works utilizing these methods have estimated the EIE for most non-organic NO_y compounds (Walters and Michalski, 2015). For KIE, in addition to molecular constants, the transition state vibrational frequencies are also needed. The only ¹⁵N KIE calculation to date for an NO_y compound is for the KIE for the NO + O₃ reaction (Walters and Michalski, 2016).

These EIE and KIE values have been incorporated in i_NRACM in this study (Table S2a–c). If there is no isotope effect associated with any of the NO_y reactions, then α is set to be equal to 1. The ¹⁵N isotopologue rate constant for any reaction is then α¹⁴k, where ¹⁴k is the rate constant for any ¹⁴N reaction in RACM, and these are given in Table S2a–f. It is useful to define the magnitude of EIE and KIE in the same per mill (‰) notation used to quantify a δ¹⁵N values by defining an enrichment factor ε (‰) = (α−1)1000. For example, in the NO_x isotope exchange equilibrium mentioned above, the ${\mathit{\epsilon }}_{\mathrm{NO}/{\mathrm{NO}}_{\mathrm{2}}}=-\mathrm{35.7}$ ‰. This means that the ${}^{\mathrm{15}}\mathrm{NO}/\mathrm{NO}$ ratio would be 35.7 ‰ smaller than the ${}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}/{\mathrm{NO}}_{\mathrm{2}}$ ratio if the isotopes in two gases were equilibrated (Table S2b).

PHIFE is the relative change in photolysis rates of isotopologues due to the substitution of a heavier isotope (Yung and Miller, 1997). In the atmospheric N cycle, NO₂, NO₃, N₂O₅, and HONO readily undergo photolysis at wavelengths of light that penetrate into the troposphere. The PHIFE can be estimated using a simple zero-point energy shift model (ΔZPE). In this approximation, the absorption spectra of the heavier isotopologue is generated by applying a uniform blue shift (equal to ΔZPE) to the measured spectral absorbance of the light (major) isotopologue (Blake et al., 2003; Liang et al., 2004; Miller and Yung, 2000). This results in isotopic fractionation because the wavelength-dependent (λ-dependent) photolysis rate constant (J(λ)) is dependent on the convolution of the absorption cross section (σ(λ)), actinic flux (F(λ)), and quantum yield (ϕ(λ)) (Eq. 2):

$$\begin{array}{}\text{(2)}& {}^{x}J\left(\mathit{\lambda }\right){=}^x\mathit{\sigma }\left(\mathit{\lambda }\right)F\left(\mathit{\lambda }\right)\mathit{\varphi }\left(\mathit{\lambda }\right).\end{array}$$

The overall photolysis rate constant (^zJ) can be calculated by integrating σ, F, and ϕ over a range of wavelengths that can cause dissociation (λ₁ and λ₂):

$$\begin{array}{}\text{(3)}& {}^{x}J=\underset{{\mathit{\lambda }}_{\mathrm{1}}}{\overset{{\mathit{\lambda }}_{\mathrm{2}}}{\int }}{}^x\mathit{\sigma }\left(\mathit{\lambda }\right)F\left(\mathit{\lambda }\right)\mathit{\varphi }\left(\mathit{\lambda }\right)\mathrm{d}\mathit{\lambda }.\end{array}$$

The N isotopologue fractionation (α) resulting from photolysis (of NO₂ isotopologues) is calculated by Eq. (4).

$$\begin{array}{}\text{(4)}& {\mathit{\alpha }}_{\mathrm{47}/\mathrm{46}}={\displaystyle \frac{{}^{\mathrm{47}}J}{{}^{\mathrm{46}}J}}\end{array}$$

It is important to note that there are limitations in the ΔZPE-shift model (Blake et al., 2003; Liang et al., 2004; Miller and Yung, 2000). These include the failure to account for changes in the shape and intensity of absorption spectra upon isotopic substitution, and the same quantum yield (as a function of wavelength) is assumed for all isotopologues. Despite these limitations, this approach should still give a rough estimate of photolytic fractionation until experimentally determined PHIFEs become available (Blake et al., 2003; Liang et al., 2004; Miller and Yung, 2000).

Isotopologues partition differently between phases giving rise to the VPIE. This is most notable in gas–liquid systems (Van Hook et al., 2001) but can also occur in gas–solid equilibrium. Both of these may ultimately be important for understanding δ¹⁵N variability in NO_y compounds. For example, solid–gas VPIE may be relevant for the HNO_3(g)+ NH_3(g) $\leftarrow \to$ NH₄NO_3(s) reaction, whose temperature-dependent equilibrium can shift dramatically diurnally (Morino et al., 2006) and seasonally (Paulot et al., 2016). It is likely that this VPIE will result in the particle-phase NO${}_{\mathrm{3}}^{-}$ having a different δ¹⁵N value compared to the gas-phase HNO₃ (Heaton, 1987). Additionally, possible VPIE occurring during wet and dry deposition, such as HNO_3(g) → HNO_3(aq), may be relevant for δ¹⁵N variations NO${}_{\mathrm{3}}^{-}$ in precipitation (Freyer et al., 1993). Multiphase reactions are not included in RACM since it is only concerned with gas-phase reactions. These effects may be important for accurate δ¹⁵N predictions and should be addressed in more complex models, but this is a limitation in any “gas-phase-only” photochemical box model. Similarly, NO_y aqueous-phase reactions, such as 2NO₂ + H₂O → HNO₃ + HNO₂, are not included in RACM, which may limit i_NRACM's ability to accurately predict the δ¹⁵N values of dissolved NO${}_{\mathrm{3}}^{-}$ in rainfall samples.

Figure 1 The time evolution of δ¹⁵N values of NO, NO₂, HONO, and HNO₃, caused by the NO₃+ NO → NO₂+ NO₂ reaction (Reaction R51, 51a). This reaction only induces a δ¹⁵N variation of ±0.005 ‰ in the relevant compounds. Thus, this reaction is considered irrelevant and i_NRACM sets R51a α=1.0.

2.3 Sensitivity analysis: determining the “reaction relevance” of NO_y isotopologues

The objective of the i_NRACM model is to make predictions about the temporal and spatial variation of δ¹⁵N values in various N compounds caused by EIE, KIE, and PHIFE, and compare them to observations. Currently, the δ¹⁵N observations are largely limited to HNO₃, as either particulate or dissolved NO${}_{\mathrm{3}}^{-}$, but there are a few recent measurements of the δ¹⁵N values of NO_x (Walters et al., 2018) and HONO (Chai and Hastings, 2018). The δ¹⁵N values of organic nitrates and PAN may be made in the not-so-distant future, but there are no published data to date. Thus, a given isotopologue reaction pair in i_NRACM was considered “relevant” if it significantly changed the δ¹⁵N value (±1 ‰) of NO_x, HONO, or HNO₃. This relevance was determined by conducting a sensitivity analysis on the PHIFE, KIE, and EIE effects for all N reactions. This was done by arbitrarily setting α=0.98 ($\mathit{\epsilon }=-\mathrm{20}$ ‰) for one isotopologue reaction and α=1.0 for all others, then running a test case. This test case is a 5 d simulation, beginning at 03:00 local time (LT) on 1 March 2007, and simulates mid-latitude suburban chemistry using the trace gas and meteorology parameters given in Table S3a and b. This simulation was repeated 96 times until every N-containing reaction was tested. For example, NO_x, HONO, or HNO₃ δ¹⁵N values are not sensitive to Reaction (R51) (Fig. 1). The following section discusses which i_NRACM reactions are relevant and the approaches used to determine the appropriate α values for those reactions. These simulations were also used to test whether i_NRACM achieves N isotope mass balance via Σ¹⁵N/Σ¹⁴N, where the sums are the ending abundances of all N compounds. This resulted in δ¹⁵N = 0 for all simulations. We also tested whether the addition of ¹⁵N isotopologues had any effect on the RACM's predictions of trace gases over time. Plots of mixing ratios of trace gases such as HNO₃ and O₃ predicted by RACM versus those i_NRACM run under the same conditions (see Stockwell's 24 simulation tests) yield a slope of 1 with an R²>0.99, which is expected since the addition of ¹⁵N compounds is only about 0.3 % of total NO_x and thus should not differ from the RACM predictions.

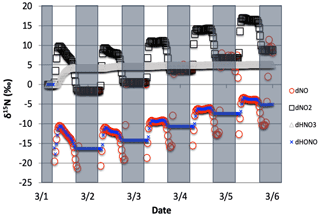
Figure 2 The time evolution of δ¹⁵N values of NO, NO₂, HNO₃, and HONO caused by PHIFE during NO₂ photolysis.

2.3.1 PHIFE relevant in the i_NRACM mechanism

Only 1 of the 6 photolysis reactions involving N compounds was found to be relevant. NO₂ photolysis (Reaction R1) had a significant impact on the δ¹⁵N value of NO_x, HONO, and HNO₃ (Fig. 2). The initial difference between the δ¹⁵N of NO and NO₂ values is roughly equal to the arbitrarily set −20 ‰ enrichment factor. The nature of the diurnal oscillation in δ¹⁵N values on the three relevant NO_y compounds and the dampening effect over time will be discussed in the results section.

Figure 3 The time evolution of δ¹⁵N values of NO₃, and N₂O₅ caused by PHIFE during NO₃ photolysis.

When there is sufficient photolysis of any single NO_y compound, then the δ¹⁵N value of that compound tends to significantly change, but often neither the HNO₃, HONO, nor NO_x δ¹⁵N values are affected. For example, the arbitrary α for NO₃ photolysis (Reactions R7 and R8) alters the δ¹⁵N value of HNO₃ and NO_x by less than 0.1 ‰ (not shown), but it induces large diurnal changes in the δ¹⁵N value of NO₃ and N₂O₅, with sharp transitions occurring during sunrise and sunset (Fig. 3). This is easily understood. For our test case, during the day ¹⁵NO₃ would be left behind because ¹⁴NO₃ is preferentially being photolyzed. The daytime N₂O₅ formed from this NO₃ (positive δ¹⁵N) and NO₂ (δ¹⁵N ∼0) thus has a δ¹⁵N value halfway between these two reactants (isotope mass balance). However, there is so little NO₃ and N₂O₅ during the day that essentially no HNO₃ is being formed through these precursors, and the NO₃ PHIFE is not manifested in the NO_x or HNO₃ δ¹⁵N value. During the night, photolysis and the PHIFE ceases and any NO₃ and N₂O₅ formed by NO₂ oxidation have δ¹⁵N values equal to the NO₂.

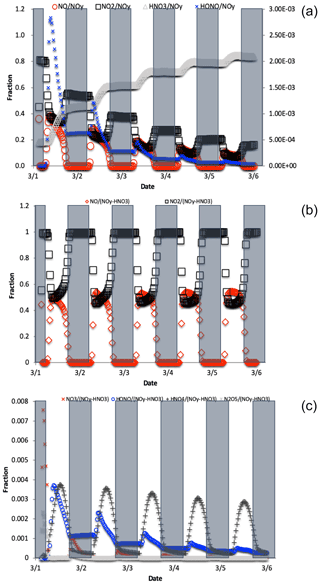
Figure 4 The change in f_NO, $f_{{\mathrm{NO}}_{\mathrm{2}}}$, $f_{{\mathrm{NO}}_{\mathrm{3}}}$, and f_HONO (right axis) over the 5 d simulation shows the transition from NO_y as mostly NO_x to predominately HNO₃ (a). For reactive NO_y (NO_y–HNO₃) large diurnal changes in f_NO and $f_{{\mathrm{NO}}_{\mathrm{2}}}$ (b) caused by photolysis minimize the other $f_{{\mathrm{NO}}_y}$ values, none of which exceeds 0.01 (c).

NO_x, HONO, and HNO₃ are not sensitive to the other NO_y photolysis reactions because of this isotope mass balance effect.

$$\begin{array}{}\text{(5)}& {\mathit{\delta }}^{\mathrm{15}}{\mathrm{N}}_{{\mathrm{NO}}_y}=\mathrm{\Sigma }{f}_{{\mathrm{NO}}_{y}i}\cdot \mathit{\delta }{}^{\mathrm{15}}{\mathrm{N}}_{{\mathrm{NO}}_y\mathrm{i}},\end{array}$$

where $f_{{\mathrm{NO}}_{y}i}$ is the mole fraction of any NO_yi compound relative to total NO_y, δ¹⁵N${}_{{\mathrm{NO}}_{y}i}$ is the δ¹⁵N value of that compound, and δ¹⁵N${}_{{\mathrm{NO}}_y}$ is the value of total N, which in these simulations is arbitrarily set to 0 ‰. For an $\mathit{\epsilon }=-\mathrm{20}$ ‰ and a threshold of “importance” set to ±1 ‰, isotope mass balance requires that $f_{{\mathrm{NO}}_{y}i}>\mathrm{0.05}$. Only NO, NO₂, HONO, and HNO₃ compounds meet this threshold (Fig. 4). All other $f_{{\mathrm{NO}}_{y}i}$ values are an order of magnitude smaller, the largest being $f_{{\mathrm{HNO}}_{\mathrm{4}}}$, and it only reaches a maximum value of 0.005. By the end of the second simulation day the $f_{{\mathrm{HNO}}_{\mathrm{3}}}$ has approached 1 and effectively minimizes the other $f_{{\mathrm{NO}}_{y}i}$ values because it is the only stable N compound, as the other NO_y compounds are very photochemically active. If we exclude this buildup in HNO₃ from the sum of NO_y, then f_NO and $f_{{\mathrm{NO}}_{\mathrm{2}}}$ (and HONO during some hours; see discussion) become the dominant fractions (Fig. 4), and they control the other $f_{{\mathrm{NO}}_{y}i}$. Even under this constraint, the $f_{{\mathrm{HNO}}_{\mathrm{4}}}$ only reaches 0.001 (Fig. 4). Thus, in i_NRACM, the α values of α_R4−α_R8 were set to be equal to 1 and only the α_R1 was assigned a non-1 value, which was determined using a PHIFE theory (discussed below).

2.3.2 KIE relevant in i_NRACM mechanism

The KIE for 12 N-containing compounds and their 96 reactions were evaluated using the same sensitivity analysis. The vast majority of reactions had little influence on the δ¹⁵N values of NO_x, HONO, and HNO₃ (Fig. 1). Similar to the photolysis sensitivity, either reaction proximity or isotope mass balance were controlling δ¹⁵N relevance. For example, NO₂ + OH is a reaction that directly produces a significant fraction of HNO₃ and therefore Reaction (R39) is relevant in the i_NRACM mechanism. In contrast, Reaction (R95) produces very little HNO₃ so it has a negligible influence on the predicted HNO₃ δ¹⁵N value. Therefore, the only relevant KIE reactions that have α≠1 in the i_NRACM mechanism are Reactions (R39), (R91)–(R97), and (R48) (Table S2b).

2.3.3 EIE relevant in i_NRACM mechanism

While some EIEs are naturally handled in the i_NRACM mechanism, such as the NO₂–NO₃–N₂O₅ equilibrium, other potentially important N isotope exchange reactions are not directly expressed in RACM and must be considered. From a thermodynamic perspective, the EIE for any two N-containing compounds can be calculated. The rate at which these compounds can achieve equilibrium, however, needs careful consideration. For example, the EIE for the isotope exchange reaction NO + ¹⁵HNO₃ $\leftarrow {\to }^{\mathrm{15}}$NO + HNO₃ has been calculated and measured (Brown and Begun, 1959). Yet, steric considerations would suggest it would be very improbable for a gas-phase reaction pathway or transition state to exist where two O atoms and a hydrogen atom from a HNO₃ could quickly migrate to a NO molecule during a collision. The result is that isotope exchange for this gas-phase reaction is likely kinetically too slow to be relevant but is valid in a highly concentrated liquid phase (Brown and Begun, 1959). The larger the N-containing molecule the more difficult it is to envision gas-phase EIE occurring on a timescale comparable to the residence time tropospheric N of about a week. On the other hand, the isotope exchange reaction NO + ¹⁵NO₂ $\leftarrow {\to }^{\mathrm{15}}$NO + NO₂ rapidly occurs (Sharma et al., 1970) because it can form an ONONO (N₂O₃) stable intermediate. As such, i_NRACM only considers N isotope equilibrium between NO, NO₂, NO₃, and N₂O₅. Since the latter three compounds are already chemically equilibrated in RACM, they are by default isotopically equilibrated in i_NRACM. Therefore, the only new isotope exchange reaction added to i_NRACM was NO + ¹⁵NO${}_{\mathrm{2}}\leftarrow {\to }^{\mathrm{15}}$NO + NO₂ (Reactions R238, R238a).

2.4 Isotopologue fraction factors (α) used in i_NRACM

In this section we discuss the methodology used to determine the values for the relevant PHIFE, KIE, and EIE. These are Reactions (R1), (R39), (R48), (R91)–(R97), and (R238).

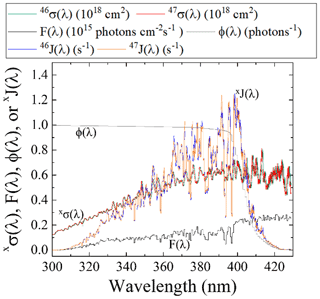
Figure 5 Literature-reported ⁴⁶σ(λ) (Vandaele et al., 2002) F(λ) (at SZA of 60^∘; TUV model), and NO₂ϕ(λ) (Roehl et al., 1994) and calculated ⁴⁷σ(λ) derived from the ZPE shift model for wavelengths relevant for tropospheric conditions for NO₂ photolysis. From these parameters, both ⁴⁶J(λ) and ⁴⁷J(λ) have been calculated (Eq. 2).

2.4.1 PHIFE-derived α used in the i_NRACM mechanism

The PHIFE for Reaction (R1) was calculated using an existing NO₂ experimental photolysis cross section of ¹⁴NO₂ for tropospheric relevant wavelengths (300 to 450 nm) (Vandaele et al., 2002). Using the experimentally determined ΔZPE for the ¹⁵NO₂ isotopologue of 29.79 cm⁻¹ (Michalski et al., 2004), the ⁴⁷σ(λ) was blue shifted by roughly 0.3 nm from the experimentally measured ⁴⁶σ(λ) (Vandaele et al., 2002) (Fig. 5). The wavelength-dependent actinic flux, F(λ), was taken from the TUV model (NCAR) for solar zenith angles from 0 to 90^∘ in 15^∘ increments. The ϕ(λ) values were taken from experimental data at 298 K (Roehl et al., 1994), and it was assumed that there is no significant quantum yield isotope effect. Based on these assumptions the ⁴⁶J(λ) and ⁴⁷J(λ) values were calculated (Fig. 5). An important feature of NO₂ the wavelength-dependent J includes a peak near 390–400 nm that subsequently decreases at longer wavelengths until NO₂ photolysis ceases beyond 420 nm due to a ϕ=0 (Roehl et al., 1994). Overall, the NO₂ PHIFE α value was found to be consistent for the wide range of solar zenith angles, ranging between 1.002 and 1.0042 with higher values occurring at lower solar zenith angles. We used an α=1.0042 for daylight hours.

2.4.2 KIE-derived α used in the i_NRACM mechanism

KIE for the NO + O₃ reaction

The ¹⁵α₄₈ for the NO + O₃→ NO₂ + O₂ reaction was determined by ab initio calculations (Walters and Michalski, 2016). Generally, in a normal KIE the heavy ¹⁵NO would react with O₃ slower than the light ¹⁴NO, which is consistent with the calculated effect; however, it is relatively small ($\mathit{\epsilon }=-\mathrm{6.7}$ ‰ at 298 K). The ¹⁵α₄₈ was determined to have the following temperature-dependent relationship (Walters and Michalski, 2016) over the temperature range of 220 to 320 K (Eq. 6):

$$\begin{array}{}\text{(6)}& {\mathit{\alpha }}_{\mathrm{48}}=(\mathrm{0.9822}\cdot \text{exp}(\mathrm{3.3523}/T).\end{array}$$

KIE for the NO₃ + VOC reactions

The most influential reactions that impacted the δ¹⁵N of HNO₃ were the three reaction pathways that generate HNO₃. This is because the isotope effect associated with this last step is largely retained in the product HNO₃ because photolysis of HNO₃ back into photochemically active compounds that could re-scramble N isotopes is slow, effectively “locking in” these final isotope effects. Two gas-phase reaction groups are important for HNO₃ production. Nitric acid is produced mainly by Reaction (R39) during the daytime (Seinfeld and Pandis, 1998) but this reaction is treated as an EIE as discussed below in the EIE section. During the nighttime, when the photolysis sink for NO₃ vanishes, NO₃ can react with VOCs to form HNO₃ via hydrogen abstraction reactions (Atkinson, 2000). Any individual NO₃ + VOC reaction had a small “relevance” for the δ¹⁵N values of NO_x, and HNO₃, but given there are 7 such reactions (Reactions R91–R97) their sum may be important.

The KIE for each of the NO₃ + VOC → HNO₃ reaction (Reactions R91–R97) was determined by assuming collisional frequency was the key KIE factor in such reactions. In these reactions (Reactions R91–R97) NO₃ abstracts hydrogen from a hydrocarbon, acting through a transition state involving the oxygen atoms in the nitrate radical C–H–ONO₂. Since N is not directly participating in the bond formation it is classified as a secondary KIE (Wolfsberg, 1960). Secondary KIEs are typically much smaller than primary KIEs that occur at bond-breaking and bond-forming positions within a molecule (Wolfsberg, 1960). Therefore, we assumed that the secondary KIE was negligible and did not factor into the α values for these 7 reactions. On the other hand, isotope substitution does change the relative rate of collisions for N isotopologues because of the change in molecular mass. The collisional frequency (Eq. 7) for any of the NO₃ + VOC reaction pairs was calculated assuming a hard sphere approximation via

$$\begin{array}{}\text{(7)}& A={\left[{\displaystyle \frac{\mathrm{8}\mathrm{kT}}{\mathit{\pi }\mathit{\mu }}}\right]}^{\mathrm{1}/\mathrm{2}}\mathit{\pi }{d}^{\mathrm{2}},\end{array}$$

where μ is the reduced mass of either NO₃ or ¹⁵NO₃ and the specific hydrocarbon in a given reaction (Reactions R91–R97). When taking the isotopologue collision ratio, the constants, collision cross section (d²), and temperature cancel out giving a temperature-independent KIE of

$$\begin{array}{}\text{(8)}& \mathit{\alpha }={\displaystyle \frac{k_{\mathrm{15}}}{k_{\mathrm{14}}}}={\displaystyle \frac{A_{\mathrm{15}}}{A_{\mathrm{14}}}}=\sqrt{{\displaystyle \frac{{\mathit{\mu }}_{\mathrm{15}}}{{\mathit{\mu }}_{\mathrm{14}}}}}.\end{array}$$

The α for each NO₃ + VOC reaction (Reactions R91–R97) is calculated using the hydrocarbon mass (Table S1b) and the NO₃ isotopologue masses (62, 63 amu) and using Eq. (8).

2.4.3 EIE-derived α used in the i_NRACM mechanism

EIE of NO + NO₂ exchange

The NO + NO₂ exchange was added to i_NRACM by defining a forward and reverse reaction (Reactions R238, R238a) and an equilibrium constant $K_{\mathrm{238}}=k_{\mathrm{238}}/k_{\mathrm{238}a}=\mathit{\alpha }$. The forward rate constant (k₂₃₈) was based on the NO-NO₂ isotope exchange rate determined by Sharma et al. (1970) (3.6×10¹⁴ cm³ s⁻¹ molecule⁻¹). The reverse rate was calculated using $k_{\mathrm{238}}=k_{\mathrm{238}a}/{\mathit{\alpha }}_{\mathrm{238}}$. The temperature-dependence of EIE of NO + NO₂ exchange (Eq. 9) was calculated using quantum mechanical techniques (Walters and Michalski, 2015) that matched well with recent experimental values (Walters et al., 2016).

$$\begin{array}{}\text{(9)}& {\mathit{\alpha }}_{\mathrm{238}}=\mathrm{0.9771}\cdot \text{exp}(\mathrm{18.467}/T)\end{array}$$

EIE used in the NO₂+OH reaction

The ¹⁵α₃₉ for the NO₂ + OH + M → HNO₃ reaction (R39) was determined by assuming equilibrium between NO₂ and HNO₃. The third body and the negative temperature dependence of the rate constant shows that, similar to O₃ formation, this reaction is an association reaction (Golden and Smith, 2000). It proceeds through an excited intermediate, *HNO₃, that can undergo collisional deactivation by a third body M (Eq. 10).

$$\begin{array}{}\text{(10)}& {\mathrm{NO}}_{\mathrm{2}}+\mathrm{OH}\leftarrow k_{\mathrm{r}}{k}_{\mathrm{f}}{\to }^{\ast }{\mathrm{HNO}}_{\mathrm{3}}{k}_{\mathrm{d}}\to {\mathrm{HNO}}_{\mathrm{3}},\end{array}$$

in which k_f and k_r are the forward and reverse rate constants for the association step, and k_q is the rate constant for collisional quenching and deactivation of the activated complex. We have calculated that $k_{\mathrm{r}}/k_{\mathrm{q}}$ is on the order of 5.5 (see SA), and thus the assumption about reactant-complex isotopic equilibrium appears to be valid since only a single decomposition would cause isotopic equilibrium. The HNO₃ production rate constant is then k_fk_d[M]/k_r=K_eqk_d[M]. This general form can be used to write two isotopologue equilibrium constants K:

$$\begin{array}{}\text{(11)}& {\displaystyle }{K}_{\mathrm{39}}={[}^{*}{\mathrm{HNO}}_{\mathrm{3}}]/(\left[{\mathrm{NO}}_{\mathrm{2}}\right]\left[\mathrm{OH}\right])=k_{\mathrm{39}\mathrm{f}/}{k}_{\mathrm{39}\mathrm{r}},\text{(12)}& {\displaystyle }{K}_{\mathrm{39}\mathrm{a}}={[}^{*}{\mathrm{H}}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{3}}]/(\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]\left[\mathrm{OH}\right])=k_{\mathrm{39}\mathrm{af}}/k_{\mathrm{39}\mathrm{ar}}.\end{array}$$

Since •OH is not participating in the N isotope chemistry, these two EIEs effectively reduce the isotope chemistry to the temperature-dependent ¹⁵N EIE:

$$\begin{array}{}\text{(13)}& {\displaystyle }{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}{+}^{*}{\mathrm{HNO}}_{\mathrm{3}}\leftarrow \to {\mathrm{NO}}_{\mathrm{2}}{+}^{*}{\mathrm{H}}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{3}},\text{(14)}& {\displaystyle }{K}_{\mathrm{39}\mathrm{a}}/K_{\mathrm{39}}={\mathit{\alpha }}_{{\mathrm{HNO}}_{\mathrm{3}}/{\mathrm{NO}}_{\mathrm{2}}}={\mathit{\beta }}_{{\mathrm{HNO}}_{\mathrm{3}}}/{\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{2}}}.\end{array}$$

The fundamental vibration frequencies for HNO₃* were taken to be the same as ground-state HNO₃, similar to RRKM theory approaches used to calculate the uni-molecular decay rate of HNO₃* (Golden and Smith, 2000). The temperature-dependent ${\mathit{\beta }}_{{\mathrm{HNO}}_{\mathrm{3}}}$ and ${\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{2}}}$ values for this exchange were taken from Walters and Michalski (2015). Since the reaction has a negative activation energy and has a fairly rapid rate constant at 101 kPa (1×10¹¹ cm⁻³ s⁻¹) and the isotope effect due to the collisional deactivation frequency (Eq. 7) is minimal (∼2 ‰) compared to the equilibrium effect (∼40 ‰), the deactivation rate constants k_d were set to be equal ($k_{\mathrm{d}\mathrm{14}}/k_{\mathrm{d}\mathrm{15}}=\mathrm{1}$). Setting k_r14=k_r15, and using the ${\mathit{\alpha }}_{{\mathrm{HNO}}_{\mathrm{3}}/{\mathrm{NO}}_{\mathrm{2}}}$ equilibrium value, k_39a for the ¹⁵NO₂+ OH → H¹⁵NO₃ reaction is

$$\begin{array}{}\text{(15)}& K_{\mathrm{39}\mathrm{a}}={\mathit{\alpha }}_{{\mathrm{HNO}}_{\mathrm{3}}/{\mathrm{NO}}_{\mathrm{2}}}\left(K_{\mathrm{39}}\right).\end{array}$$

The temperature dependence of ${\mathit{\alpha }}_{{\mathrm{HNO}}_{\mathrm{3}}/{\mathrm{NO}}_{\mathrm{2}}}$ is derived from the tables in Walters and Michalski (2015) and α₃₉ is then

$$\begin{array}{}\text{(16)}& {\mathit{\alpha }}_{\mathrm{39}}=(\mathrm{0.973}\cdot \text{exp}(\mathrm{19.743}/T).\end{array}$$

For typical tropospheric temperatures the ${\mathit{\alpha }}_{{\mathrm{HNO}}_{\mathrm{3}}/{\mathrm{NO}}_{\mathrm{2}}}\mathrm{1.040}$ suggesting the δ¹⁵N of HNO₃ produced by the NO₂+ OH reaction will be +40 ‰ relative to tropospheric NO₂. This α value is larger and opposite the sign of the ¹⁵α=0.9971 assumed by Freyer (1991). Freyer's α was approximated by using the reduced mass of the OH–NO₂ activated complex. There two problems with this approach. First, the activation complex's reduced mass approximation should be viewed in terms of the decomposition rate constant, not the product formation rate constant as assumed by Freyer, because transition state theory assumes equilibrium between the stable reactants and the transition state (Bigeleisen and Wolfsberg, 1958; Wolfsberg et al., 2010). In other words, Freyer's α=0.9971 should indicate that the ¹⁵NO₂-OH decomposes more slowly than ¹⁴NO₂-OH and is therefore more likely to form HNO₃ at +2.9 ‰ (not −2.9 ‰ determined in Freyer). Secondly, the reduced mass approximation of the complex pair ignores the thermodynamic contribution of the reactants and the vibrations in the transition state other than the bond forming (imaginary) vibration. Our approach overcomes both of these assumptions and incorporates the temperature dependence of the EIE for this reaction.

EIE used in heterogeneous reactions of N₂O₅

During the nighttime, the heterogeneous HNO₃ formation pathway becomes important (Chang et al., 2011; Dentener and Crutzen, 1993; Riemer et al., 2003). During the night, NO is nearly completely oxidized to NO₂ leading to the buildup of the NO₃ radical (Reaction R48), the formation of N₂O₅ (Reaction R53), and heterogeneous N₂O₅ hydrolysis becomes a major source of HNO₃ production (discussed below). This is particularly true in regions that have high NO_x mixing ratios and large aerosol surface areas such as urban centers (Chang et al., 2011; Riemer et al., 2003). In order to assess the ¹⁵N partitioning of this reaction pathway, both EIE and KIE were considered.

It was assumed that the fractionation factor for the N₂O₅ → 2HNO₃ reaction was mainly controlled by nighttime equilibrium between N₂O₅ and ${\mathrm{NO}}_{\mathrm{2}}/{\mathrm{NO}}_{\mathrm{3}}$ (Reactions R53, R54). When factoring the isotopologue dynamics, this equilibrium can be viewed as an EIE via

$$\begin{array}{}\text{(17)}& {}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{NO}}_{\mathrm{3}}\leftarrow \to {\mathrm{O}}_{\mathrm{2}}^{\mathrm{15}}\mathrm{N}-\mathrm{O}-{\mathrm{NO}}_{\mathrm{2}}\leftarrow \to {\mathrm{NO}}_{\mathrm{2}}+{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{3}},\end{array}$$

where ¹⁵N₂O₅ is represented as the transition state O${}_{\mathrm{2}}^{\mathrm{15}}$N–O–NO₂ to highlight the relative ease of N isotope exchange via oxygen migration during N₂O₅ formation and decomposition. The symmetry of ¹⁵NNO₅ and N¹⁵NO₅ is also why they were not treated as isotopomers since they are structurally identical.

The N₂O₅ equilibrium in the RACM model is dealt with as a forward Reaction (R53) (k₅₃) and a decomposition Reaction (R54) (k₅₄) that are derived from the measured equilibrium constant (K₅₃) = ($k_{\mathrm{53}}/k_{\mathrm{54}}$). In i_NRACM the N₂O₅ isotopologue has two formation pathways, with two forward rate constants (k_53 a,b) and two decomposition rate constants (k_54a,b) that were used to write their respective equilibrium constants K:

$$\begin{array}{}\text{(18)}& {\displaystyle }{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{NO}}_{\mathrm{3}}\leftarrow \to {}^{\mathrm{15}}{\mathrm{NNO}}_{\mathrm{5}}(K_{\mathrm{53}\mathrm{a}}=k_{\mathrm{53}\mathrm{a}}/k_{\mathrm{54}\mathrm{a}}),\text{(19)}& {\displaystyle }{\mathrm{NO}}_{\mathrm{2}}+{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{3}}\leftarrow \to {}^{\mathrm{15}}{\mathrm{NNO}}_{\mathrm{5}}(K_{\mathrm{53}\mathrm{b}}=k_{\mathrm{53}\mathrm{b}}/k_{\mathrm{54}\mathrm{b}}).\end{array}$$

Dividing K_53a and K_53b by K₅₃ yields isotopologue product and reactant ratios that can be evaluated using β(α) values from Walters and Michalski (2015). These were used to determine the α value for the N₂O₅ isotopologue equilibrium, which is simply a function of the formation and decomposition rate constants and temperature:

$$\begin{array}{}\text{(20)}& {\displaystyle }\begin{array}{rl}{K}_{\mathrm{53}\mathrm{a}}/K_{\mathrm{53}}& =\left({}^{\mathrm{15}}{\mathrm{NNO}}_{\mathrm{5}}/{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}\right)\left({\mathrm{NO}}_{\mathrm{2}}{/}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right)\left({\mathrm{NO}}_{\mathrm{3}}/{\mathrm{NO}}_{\mathrm{3}}\right)\\ & ={\mathit{\beta }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}/{\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{2}}}={\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}/{\mathrm{NO}}_{\mathrm{2}}}\\ & =k_{\mathrm{53}\mathrm{a}}/k_{\mathrm{53}}\times k_{\mathrm{54}}/k_{\mathrm{54}\mathrm{a}},\end{array}\text{(21)}& {\displaystyle }\begin{array}{rl}{K}_{\mathrm{53}\mathrm{b}}/K_{\mathrm{53}}& =\left({}^{\mathrm{15}}{\mathrm{NNO}}_{\mathrm{5}}/{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}\right)\left({\mathrm{NO}}_{\mathrm{3}}{/}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{3}}\right)\left({\mathrm{NO}}_{\mathrm{2}}/{\mathrm{NO}}_{\mathrm{2}}\right)\\ & ={\mathit{\beta }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}/{\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{3}}}={\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}/{\mathrm{NO}}_{\mathrm{3}}}\\ & =k_{\mathrm{53}\mathrm{b}}/k_{\mathrm{53}}\times k_{\mathrm{54}}/k_{\mathrm{54}\mathrm{b}}.\end{array}\end{array}$$

The N₂O₅ decomposition rate constants were arbitrarily set to be equal ($k_{\mathrm{54}}=k_{\mathrm{54}\mathrm{a}}=k_{\mathrm{54}\mathrm{b}}$), and the decomposition rate constants were then derived using the temperature-dependent α values:

$$\begin{array}{}\text{(22)}& {\displaystyle }{k}_{\mathrm{53}\mathrm{a}}=k_{\mathrm{53}}\left({\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}/{\mathrm{NO}}_{\mathrm{2}}}\right){\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}/{\mathrm{NO}}_{\mathrm{2}}}=\mathrm{1.0266}\left(\mathrm{298}\mathrm{K}\right),\text{(23)}& {\displaystyle }{k}_{\mathrm{53}\mathrm{b}}=k_{\mathrm{53}}\left({\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}/{\mathrm{NO}}_{\mathrm{3}}}\right){\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}/{\mathrm{NO}}_{\mathrm{3}}}=\mathrm{1.0309}\left(\mathrm{298}\mathrm{K}\right).\end{array}$$

The α for the doubly substituted ¹⁵N₂O₅ isotopologue was determined using $\mathit{\alpha }={\mathit{\beta }}_{{}^{\mathrm{15}}{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}/{\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\beta }}_{{\mathrm{NO}}_{\mathrm{3}}}$, and the value for ${\mathit{\beta }}_{{}^{\mathrm{15}}{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$(1.272) was approximated using the principle of the geometric mean (Bigeleisen, 1958; Snyder et al., 1999), yielding a temperature-independent α=1.057. However, the N₂O₅ system is insensitive to this α value because of the low probability of a ¹⁵N + ¹⁵N reaction ($\mathrm{1.5}\times {\mathrm{10}}^{-\mathrm{5}}$) relative to a ¹⁴N + ¹⁵N reaction ($\mathrm{4}\times {\mathrm{10}}^{-\mathrm{3}}$), and thus the small temperature dependence was also ignored.

Because RACM is a gas-phase chemical mechanism, it does not include heterogeneous reactions of N₂O₅ on aerosols, which would limit i_NRACM to accurately predict the δ¹⁵N values, particularly at night. Gas chemical mechanisms are often used in larger 1-D, 2-D, and 3-D chemical transport models that usually also include aerosol modules that calculate heterogeneous chemistry using inputs from the gas-phase chemical mechanism (i.e., N₂O₅ concentrations). However, if the objective is to use a 0-D chemical box model to simulate local chemistry the N₂O₅ heterogeneous hydrolysis will need to be included. i_NRACM was modified to use a first-order rate constant to calculate N₂O₅ heterogeneous hydrolysis (Yvon et al., 1996; Riemer et al., 2003). The rate constant is a function of N₂O₅ molecular speed (c), the N₂O₅ uptake coefficient (γ), and the aerosol surface area density S.

$$\begin{array}{}\text{(24)}& \begin{array}{rl}-{\mathrm{dN}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}/\mathrm{d}t& =\mathrm{d}\mathrm{0.5}{\mathrm{HNO}}_{\mathrm{3}}/\mathrm{d}t=k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}\left[{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}\right]\\ & =\text{R239}{k}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1}/\mathrm{4}c\mathit{\gamma }S\end{array}\end{array}$$

The $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$ values were assessed based on the different pollutant loadings and emission scenarios (Fig. 6). The $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$ was calculated as a function of γ (Anttila et al., 2006; Bertram and Thornton, 2009; Davis et al., 2008; Riemer et al., 2003, 2009) and S (Cai et al., 2017; Kuang et al., 2010; McMurry et al., 2005) values that span clean to highly polluted environments. This range yielded $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1}$, 0.1, and 0.01 for high, medium, and low polluted environments (Fig. 6).

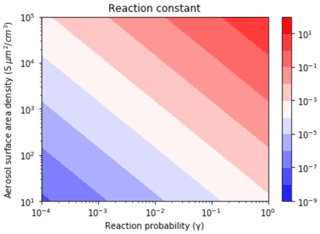
Figure 6 Contour lines of the same $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$ values as a function of γ and S values. The γ values depend on aerosol composition and range from $\mathrm{3.8}\times {\mathrm{10}}^{-\mathrm{5}}$ (relatively dry sulfuric acid) to 1 (aqueous aerosol in the winter polar stratosphere). S values are a function of aerosol number density and size distribution and range from 52 (low scavenging rate, low particle growth rate) to 1140.1 (high scavenging rate, high particle growth rate).

Only the uptake coefficient (γ) and molecular speed (c) could have a KIE during aerosol uptake of N₂O₅ (Reactions R239, R239a, R239b). The γ term was ignored because ab initio work suggests that N₂O₅ hydrolysis activates through hydrogen bonding between water molecules on the aerosol surface and O atom in the N₂O₅ (Snyder et al., 1999), making it a secondary (small) KIE for N. The c term is a function of the root of the N₂O₅ molecular mass, and when the ratio is taken there is no temperature dependence yielding ${\mathit{\alpha }}_{\mathrm{239}\mathrm{a}}=(\mathrm{108}/\mathrm{109}{)}^{\mathrm{0.5}}=\mathrm{0.995}$ and ${\mathit{\alpha }}_{\mathrm{239}\mathrm{b}}=(\mathrm{108}/\mathrm{110}{)}^{\mathrm{0.5}}=\mathrm{0.9909}$.

An online version of this i_NRACM model is available for public use at https://mygeohub.org/tools/sbox/ (last access: 30 June 2021).

2.4.4 Addition of O₃ deposition to i_NRACM

Photochemical mechanisms such as RACM are validated by comparing model predictions with observed trace gas concentration evolution in chambers studies, which has its limitations. For example, Stockwell et al. (1997) compared RACM, RADM2, and SPARC mechanisms' ability to predict trace gas concentrations (e.g., O₃, NO₂, toluene) with those observed in chamber experiments (see Stockwell et al., 1997, Figs. 3–9) and achieve good agreement between the model and experiments. These experiment–model comparisons essentially validate the rate constant assumptions in the chemical mechanism. Box models are, however, limited in their ability to predict real-world concentrations because many do not account for pollutant deposition (dry or wet) since these are handled when the mechanism is incorporated into 1-D, 2-D, and 3-D transport models. Similarly, dilution by of trace gases due to vertical (or horizontal) transport is typically not incorporated into 0-box models. This can lead to the buildup (or depletion) of key oxidants, particularly O₃ (see Fig. 6 in Stockwell et al., 1997). This in turn will significantly alter NO_x oxidation pathways, and since the δ¹⁵N in i_NRACM is effectively a function on changing oxidation pathways, this would impact i_NRACM ability to accurately predict the observations of δ¹⁵N in the real world. In order to eliminate this bias, we added a O₃ deposition reaction and adjusted the rate until O₃ mixing ratios were in line with typical suburban mixing ratios (20–30 ppb) and exhibited a typical O₃ diurnal mixing ratio variation (low nighttime and high midday) that is observed in most environments (Fig. S2). This results in simulated daytime maximum OH concentrations on the order of $\sim \mathrm{8}\times {\mathrm{10}}^{\mathrm{6}}$ molecules cm⁻³ and daytime averages of $\sim \mathrm{2}\times {\mathrm{10}}^{\mathrm{6}}$ molecules cm⁻³ (Fig. S2) that are typical of overserved concentrations in urban and suburban environments (see references in the review by Monks, 2005). This gives us confidence that i_NRACM is accurately capturing boundary layer photochemistry and can be used to predict δ¹⁵N in NO_y compounds.

2.4.5 i_NRACM simulations

A number of i_NRACM simulations were run with two different purposes. The first set of simulations iteratively changed the α values from 1 to their values discussed above. These simulations aimed at investigating the importance of each α as they aggregated together. These include photolysis only, Leighton cycle, daytime chemistry, nighttime chemistry, and full chemistry using the same test case (Table S3a–f). These were run with all α values activated but with varied initialized chemistry and primary pollutant emissions.

3 Results and discussion

It is important to first test i_NRACM by turning on and off individual relevant isotope effects and then combining their cumulative effects. This is advantageous relative to simply running the full mechanism under different pollution scenarios because it would be a challenge to disentangle which isotope effects in the full mechanism were mainly responsible for δ¹⁵N change in NO_x, HONO, or HNO₃ without such a systematic investigation. For example, it is likely that the δ¹⁵N value of NO₂ will be a significant factor in the δ¹⁵N value of HNO₃ because it is the reactant in Reactions (R39) and (R239). Thus, understanding which isotope effects control the δ¹⁵N of NO₂ helps with interpreting the δ¹⁵N value of HNO₃ and vice versa. Thus, this discussion section is divided into three sections. The first is the examination of the relevant isotope effects occurring during daytime photochemistry and their impact on NO_x, HONO, and HNO₃ δ¹⁵N values. The second is the examination of the relevant isotope effects occurring during nighttime chemistry (EIE and KIE) and their effect on NO_x, HONO, and HNO₃ δ¹⁵N values. These first two discussion sections focus mainly on the relative importance of each isotope effect when the photochemical conditions are constant. Finally, the full i_NRACM mechanism will be tested under different atmospheric conditions such as variations in trace gas concentrations, aerosol loading, and hours of sunlight. This tests how changes in photochemical oxidation pathways result in differences in the δ¹⁵N values of NO_x, HONO, and HNO₃.

3.1 The δ¹⁵N of NO_x, HONO, and HNO₃ due to daytime chemistry

The role that daytime chemistry plays in determining the δ¹⁵N values of NO_x, HONO, and HNO₃ was investigated by iteratively adding relevant fractionation factors to i_NRACM. The sensitivity of NO_x, HONO, and HNO₃ δ¹⁵N values to NO₂ photolysis (Reactions R1a) was tested. The initial trace gas concentrations and emissions were set to the 1 March test cases (Table S3a–f) and simulations were run with, and without, NO emissions. All subsequent test simulations will also use the 1 March test case in order to have a consistent comparison of δ¹⁵N values between different simulations. It is noted that the initial HNO₃ and O₃ mixing ratios are set to zero and that the start time of the simulations is 03:00 LT. The main daytime-only effects will be NO₂ photolysis (Reaction R1), O₃ oxidation (Reaction R8), and reaction OH (Reaction R39) since both photolysis and OH chemistry are only relevant during the daytime. However, NO_x isotope exchange and NO + O₃ will also play a vital role despite no being exclusively daytime reactions.

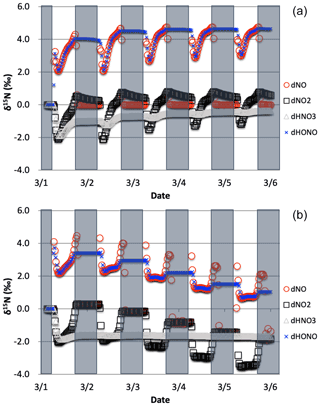
Figure 7 The δ¹⁵N values of NO, NO₂, HONO, and HNO₃ with only the photolysis isotope fractionations active. The 5 d simulation was under the conditions list in Table S3a–b. The gray boxes span night hours and the white span daytime. The top (a) is the simulation with NO_x emissions and the bottom (b) is without NO_x emissions.

3.1.1 The δ¹⁵N values of NO_x, HONO, and HNO₃ due to the photolysis only

The simulations with only R1 isotope effect activated (with NO_x emissions) shows a clear diurnal cycle in NO_x and HONO δ¹⁵N values and a multiday trend moving towards an approximate steady state for HNO₃ δ¹⁵N values, which can be explained by the PHIFE (Fig. 7a). Initially all NO_y has δ¹⁵N of zero (by default) and there is no photolysis at 03:00 LT. At sunrise the δ¹⁵N value of NO₂ goes negative and NO value positive since ¹⁵NO₂ is preferentially photolyzed (α_R1=1.0042). The difference between the δ¹⁵N values of NO and NO₂ (Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}={\mathit{\delta }}^{\mathrm{15}}$N NO − δ¹⁵N NO₂) at all times during the day is 4 ‰, which is the ε_R1a value. During the night both the NO and NO₂ δ¹⁵N values approach 0 ‰ because most NO is oxidized to NO₂ and NO emissions (0 ‰) dominate the NO nighttime budget (relative to residual day NO). Over the week-long simulation, the NO_x δ¹⁵N value slowly increases by about one per mill. This is because ¹⁵N-depleted NO₂ is converted into HNO₃ leaving the residual NO${}_x^{\mathrm{15}}$N enriched. This is also the reason for the δ¹⁵N values of HNO₃ that initially mimic the daytime NO₂ values and trends towards 0 ‰ by the end of the simulation week. The δ¹⁵N values of HONO mimic the NO values during the daytime since the main reaction pathway forming HONO is OH + NO, which peaks in the morning (∼10:00). HONO retains the evening δ¹⁵N values through the night since most of the HONO is destroyed in the afternoon via photolysis and again follows NO δ¹⁵N the next morning as its production again reaches a maximum (Fig. 7a).

The simulation without NO emissions shows a similar behavior but with some clear differences relative to the emission case. The NO_x and HONO δ¹⁵N values exhibit the same diurnal Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}=\mathrm{4}$ ‰ value. Unlike the emission case, however, the diurnal NO_x δ¹⁵N value peaks and troughs trend downward during the week-long simulation, with NO approaching 0 ‰ and NO₂ approaching −4 ‰. The HNO₃ δ¹⁵N values reach a steady-state value of roughly −1.7 ‰ after about a day and NO_x is $\sim -\mathrm{1.8}$ ‰ (Fig. 7b). This difference between the emission and non-emission case is a consequence of isotope mass balance (f_x= mole fraction of compound x relative to total NO_y).

$$\begin{array}{}\text{(25)}& \begin{array}{rl}{\mathit{\delta }}^{\mathrm{15}}{\mathrm{N}}_{\mathrm{total}}& =\mathrm{0}=f_{{\mathrm{NO}}_x}\cdot \mathit{\delta }{{}^{\mathrm{15}}\mathrm{N}}_{{\mathrm{NO}}_x}+f_{\mathrm{H},{\mathrm{NO}}_{\mathrm{3}}}\cdot \mathit{\delta }{}^{\mathrm{15}}{\mathrm{N}}_{{\mathrm{NHNO}}_{\mathrm{3}}}\\ & +f_{\mathrm{ONIT}}\cdot \mathit{\delta }{}^{\mathrm{15}}{\mathrm{N}}_{\mathrm{ONIT}}\end{array}\end{array}$$

The positive δ¹⁵N NO_y compound that effectively offsets the −1.7 ‰ in HNO₃ and −1.8 ‰ in NO_x is organic nitrate that is +2 ‰ and makes about half the NO_y pool and is roughly equal to HNO₃+ NO_x ($f_{{\mathrm{NO}}_x}=\mathrm{0.11}$, $f_{{\mathrm{HNO}}_{\mathrm{3}}}=\mathrm{0.36}$, f_ONIT=0.53). In the NO_x emission case only about 5 % of NO_y is as organic nitrate ($f_{{\mathrm{NO}}_x}=\mathrm{0.17}$, $f_{{\mathrm{HNO}}_{\mathrm{3}}}=\mathrm{0.78}$, f_ONIT=0.05), indicating a shift in oxidation pathways when NO and VOCs are emitted during the simulation relative to when they are not. In the emissions case the NO_x mixing ratios at the end of the simulation are actually slightly higher than their initial ratios, in contrast to the no-NO_x-emission case where 90 % of NO_x has been lost via oxidization into organic nitrate and HNO₃. This loss of N in the no-emission scenario effectively shuts down the oxidation chemistry. For example, the day 5 mixing ratio of O₃ is 45 ppb_v (reasonable) for the emission case but only 2 ppb_v for the non-emission case (unreasonable). Therefore, we exclude no-emission simulations for the chemistry analysis discussed in this section and restrict them to the no emission simulations to 48 h in the final test case analysis (see Sect. 4).

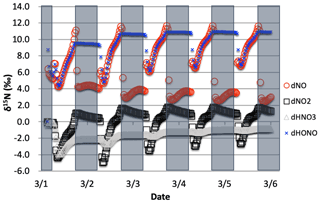
Figure 8 The δ¹⁵N values of NO_x, HONO, and HNO₃ when isotope effects associated Reactions (R1) and (R48) are combined, with NO_x emission. The 5 d simulation was under the conditions list in Table S3a and b. The diurnal patterns are reflecting the relative importance of photolysis and O₃ chemistry during the day and night.

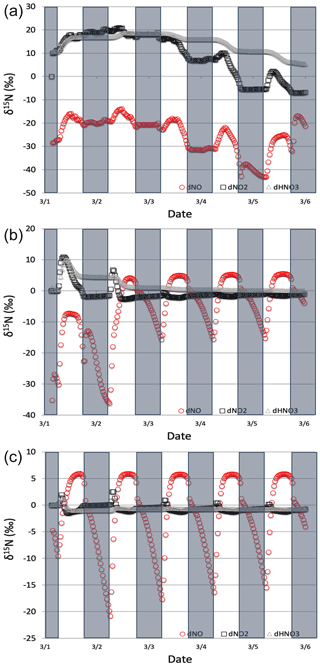
Figure 9 The δ¹⁵N values of NO_x and HNO₃ when isotope effects in Reactions (R1), (R48), and (R238) are included under high (a), medium (b), and low (c) NO_x scenarios. The 5 d simulation was under the conditions list in Table S3d–f. The NO_y δ¹⁵N values are mainly controlled by NO_x isotope exchange (Reactions R238) under high NO_x conditions and Leighton (Reactions R1 and R58) under low NO_x conditions.

3.1.2 The δ¹⁵N values of NO_x, HONO, and HNO₃ due to the combined Leighton cycle

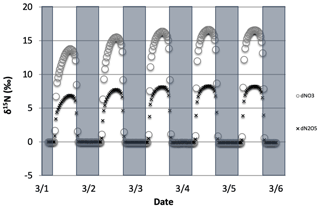
The simulations with both NO₂ photolysis (Reaction R1) and O₃+ NO (Reaction R48) isotope effects active shows similar diurnal and multiday trends to the photolysis-only simulations; they are just slightly amplified (Fig. 8). The daytime Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}$ is now ∼9.5 ‰, which is close to the additive of the two isotope effects (${\mathit{\epsilon }}_{\mathrm{48}\mathrm{a}}=-\mathrm{6.7}$ ‰, ε_R1a=4.2 ‰). This is logical since ¹⁵NO is reacting with O₃ slower than ¹⁴NO, preferentially leaving behind ¹⁵NO and thus the higher NO δ¹⁵N value. The HNO₃ δ¹⁵N values reach the mean of the daytime NO₂ δ¹⁵N values via the NO₂+ OH reaction. The slight (1 ‰) upward trends of NO_x and HNO₃ are due to isotope mass balance as detailed in the photolysis-only case. Similar to the photolysis-only case the δ¹⁵N of HONO is mimicking daytime NO δ¹⁵N values.

Figure 10 The time evolution of δ¹⁵N values of NO, NO₂, HNO₃, and HONO caused by isotope effects of Leighton reactions, NO_x isotope exchange, and NO₂+ OH reaction, with NO emission. The simulation starts from 1 March. The 5 d simulation was under the conditions list in Table S3c.

3.1.3 The δ¹⁵N values of NO_x, HONO, and HNO₃ due to the combined Leighton cycle and NO_x isotope exchange

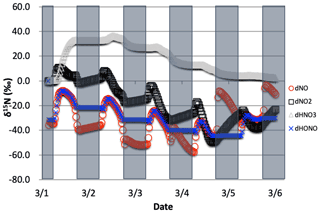
The δ¹⁵N values of NO_x produced when both the Leighton cycle and NO_x isotope exchange are active exhibit a very dynamic diurnal range that is a function of the NO_x mixing ratios. At high NO_x mixing ratios (150 ppb, one-third NO, two-thirds NO₂, Fig. 9a) the Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}$ is −40 ‰ at night as expected for NO_x isotopic equilibrium (${\mathit{\epsilon }}_{\mathrm{NO}/{\mathrm{NO}}_{\mathrm{2}}}=-\mathrm{40}$ ‰ at 298 K). During the daytime the Δδ¹⁵N${}_{{\mathrm{NO}}_x}$ shifts −30 ‰ to −35 ‰ as the photolysis and O₃ isotope effects begin to influence the Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}$. HNO₃ δ¹⁵N values during the high NO_x mixing ratio simulation initially follow the δ¹⁵N of NO₂ (via NO₂+ OH) before approaching 0 ‰, the defined NO_x source values.

At low NO_x mixing ratios (1.5 ppb, one-third NO, two-thirds NO₂, Fig. 9c) the Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}$ and HNO₃ δ¹⁵N is very different from the high NO_x simulation. The nighttime Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}$ ranges from −15 ‰ to −20 ‰ and during the daytime it is around +7 ‰, while the HNO₃ δ¹⁵N values hover around zero throughout the simulation. The difference between the NO_y δ¹⁵N values in the high and low NO_x cases can be explained as a competition between the NO_x EIE and the Leighton isotope effect. At high NO_x mixing ratios, the NO_x EIE achieves equilibrium quickly at night (Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}=-\mathrm{40}$) because the rate of NO_x isotope exchange (Reaction R238) is proportional to its concentration. In contrast, isotope exchange is slow in the low-NO_x case and the timescale to reach equilibrium is much longer. Indeed, at the low NO_x mixing ratios the nighttime equilibrium only reaches about 40 %–50 % of completion by 06:30. Afterwards sunlight begins to erase the NO_x EIE effect until around noon when the δ¹⁵N values of NO are mostly due to the Leighton effect and there is only a small contribution from EIE (about 5 %). For the intermediate NO_x mixing ratio case (15 ppb, one-third NO, two-thirds NO₂, Fig. 9b) the diurnal and week-long NO_y δ¹⁵N trends fall somewhere in between the high and low NO_x simulations.

The changes in δ¹⁵N values of HNO₃ during the 1 March simulations at differing NO_x mixing ratios can be explained in terms of HNO₃ production pathways. Over the course of day 1 the δ¹⁵N of HNO₃ mirrors that of NO₂ because of HNO₃ produced by NO₂+ OH (Reaction R39); thus the product HNO₃ δ¹⁵N values are similar to those in NO₂. This varies depending on the NO_x mixing ratio scenario for two reasons. First, as the NO_x mixing ratio gets bigger, the closer the NO_x gets to achieving the EIE and the bigger the split between NO and NO₂ δ¹⁵N values (40 ‰ versus 10 ‰ for Leighton + O₃). Second, differences in the amount of NO_x result in different $\mathrm{NO}/{\mathrm{NO}}_{\mathrm{2}}$ ratios as the simulations progress. For example, under low NO_x mixing ratios the nighttime $\mathrm{NO}/{\mathrm{NO}}_{\mathrm{2}}<\mathrm{0.001}$, which means the δ¹⁵N value of NO₂ will be close to that of total NO_x, which will be close to 0 ‰. At the same time the δ¹⁵N value of NO will be close to the fraction of the EIE achieved, which is about 50 % under low NO_x conditions, resulting in a NO δ¹⁵N of about −15 ‰. These two effects control the δ¹⁵N of NO₂ and that in turn controls the δ¹⁵N value of HNO₃. In all scenarios the diurnal cycle repeats itself over the subsequent 4 d and a greater fraction of total NO emitted has been turned into HNO₃, so that by the end of the 5 d simulation the HNO₃ δ¹⁵N values converge towards 0 ‰, the defined value of NO_x emissions in the simulations.

The modeled δ¹⁵N values of HONO also have a diurnal pattern that can also be traced to diurnal chemistry and isotope mass balance. Similar to the photolysis and photolysis + O₃ cases, the HONO δ¹⁵N values mirror the oscillation of the NO δ¹⁵N values (data not shown). This is a result of HONO production by the NO + OH reaction (Reaction R38). In contrast, the HONO δ¹⁵N values at night remain nearly constant despite the fact that the δ¹⁵N of NO is changing dramatically. This is because the absence of OH at night halts Reaction (R38) and thus HONO production ceases and the δ¹⁵N values are simply the same as the residual daytime HONO reservoir. There is a repeated minimum in HONO δ¹⁵N values occurring each morning at 07:00 LT over the subsequent 4 d. This is a result of the fact that, unlike HNO₃, HONO is effectively destroyed by photolysis (Reaction R4) and OH (Reaction R45). Thus, HONO does not build up in the model over the 5 d simulation, but rather mixing ratio peaks daily (30 ppb) at around 09:00 LT each day. This is when the HONO production–destruction rate is greatest, and its mixing ratio then deceases to a low of 2 ppt by sunset. Since the nighttime HONO, with δ¹⁵N $\sim +\mathrm{5.5}$ ‰, only contributes about 7 % (f=0.07) of the morning HONO spike, it does not greatly impact the control that NO δ¹⁵N has on the HONO δ¹⁵N value. This daily isotope effect should be contrasted with the HNO₃ δ¹⁵N trends with time. Initially HNO₃ δ¹⁵N values are influenced by NO₂ δ¹⁵N variations by NO₂-OH-HNO₃ coupling, similar to the NO-OH-HONO coupling. But since there is no significant photochemical sink of HNO₃, the control on HNO₃ δ¹⁵N values by HNO₃ accumulation increases with time, so that by day 5 the diurnal changes in NO₂ δ¹⁵N have almost no impact on the HNO₃ δ¹⁵N values (Fig. 9).

3.1.4 The δ¹⁵N values of NO_x, HONO, and HNO₃ due to the combined Leighton cycle, NO_x isotope exchange, and NO₂ + OH

The effect of the NO₂ + OH reaction has on δ¹⁵N values of NO_x and HNO₃ associated was then examined (Table S3c). Since Reaction (R39) is the last step in HNO₃ production, the instantaneous δ¹⁵N HNO₃=δ¹⁵N(NO₂) + ε₃₉, and thus the δ¹⁵N${}_{{\mathrm{HNO}}_{\mathrm{3}}}$ is initially 40 ‰ higher than the NO₂ (Fig. 10). This in turn depletes ¹⁵N in the residual NO₂ leading to more negative δ¹⁵N values in NO₂ relative to the Leighton + exchange simulations (Fig. 10). These latter two effects are still in play, as is made evident by the diurnal NO_x δ¹⁵N cycling and Δδ¹⁵N${}_{\mathrm{NO}-{\mathrm{NO}}_{\mathrm{2}}}$. As the 5 d simulation progresses, the HNO₃ δ¹⁵N value approaches 0 ‰, approaching the δ¹⁵N of NO emissions, as expected based on isotope mass balance. We point out that this convergence to the source NO_x δ¹⁵N value is much slower in this case than the Leighton and exchange cases. This highlights the importance of knowing the correct ε₄₈. If ε₃₉∼0 as suggested by Freyer (1991) then daytime the δ¹⁵N HNO₃ ≅δ¹⁵N NO₂, demonstrably lower than the ε₃₉∼40 ‰ case. In the end the average daytime δ¹⁵N value of HNO₃ for the entire simulation is about 10 ‰ higher than the δ¹⁵N of the NO_x source (here defined as 0 ‰).

3.2 The δ¹⁵N values of NO_x, HONO, and HNO₃ due to nighttime chemistry

The role that nighttime chemistry plays in determining the δ¹⁵N values of NO_x, HONO, and HNO₃ was investigated by iteratively adding relevant fractionation factors to iRACM. The nighttime chemistry effect was assessed by separating the effects of NO₃ radical chemistry and N₂O₅ heterogeneous hydrolysis. NO₃ radical chemistry is only relevant at night because of its short daytime lifetime with respect to photolysis, which keeps its daytime mixing ratios at the sub-ppt_v levels (Platt et al., 1984). At night NO₃ builds up and produces HNO₃ (Aldener et al., 2006; Finlayson-Pitts and Pitts, 1997; Horowitz et al., 1998) via reactions with hydrocarbons (Reactions R91–97). The magnitude of this isotope effect was tested by adding NO₃ to the isotope fractionation factors for Reactions (R91)–(R97) (see methods) and altering VOC emission rates to simulate clean, moderate, and extreme VOC pollution environments. Likewise, N₂O₅ only accumulates at night when it begins producing HNO₃ on aerosol surfaces (Chang et al., 2011). The magnitude of this isotope effect was tested by adding the N₂O₅ EIE (see methods) and adding the first-order N₂O₅ heterogeneous pathway (see Methods) to i_NRACM. The first-order rate constant was adjusted to simulate clean, polluted, and extreme pollution environments where aerosol surface area density largely controls the rate constant (Riemer et al., 2003; Chang et al., 2011).

3.2.1 The δ¹⁵N values of NO_x, HONO, and HNO₃ due to NO₃+ VOC reactions

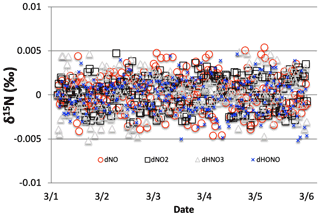
The effect on the δ¹⁵N values of NO_x, HNO₃, and HONO associated with the KIE occurring during NO₃+ VOC nighttime reactions (Reactions R91–R97) were first examined. Four simulations were run that included the isotope effects (α values in Table S4) of the Leighton cycle (Reactions R1 and R48), NO_x isotope exchange (Reaction R238), NO₂+ OH production of HNO₃ (Reaction R39), and the KIE effects (Reactions R91–R97), as well as NO emissions. The simulation tested first was the March test case (medium VOC ∼360 ppb_v). Then, two simulations were run for 1 June (extended sunlight, warm temperatures), one with high initial VOC concentrations and a high VOC emission rate (2 ppb_v h⁻¹) and one with a low emission rate of VOCs (0.4 ppb_v h⁻¹). The same two initial conditions were used in the 1 January test case to assess if the extended nighttime and cold temperatures significantly affected the NO_x of HNO₃ δ¹⁵N values produced by NO₃ radicals. The impact of NO₃ reactions on NO_y δ¹⁵N values was determined by subtracting these simulated δ¹⁵N values from those same simulations when only the Leighton cycle, exchange, and OH + NO₂ reaction were considered (Sect. 3.1).

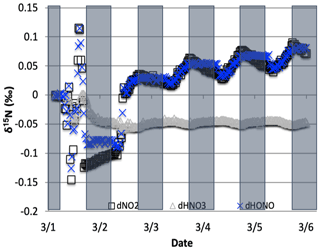
Figure 11 The difference between the δ¹⁵N values of NO₂, HONO, and HNO₃ when NO₃+ VOC → HNO₃ reactions are included and excluded (NO was omitted for clarity). The 5 d simulation was under the conditions list in Table S3e. Total VOC mixing ratios during the last day of the 1 March simulation was 550–670 ppb C.

The NO₃+ VOC KIE induced a minor diurnal pattern on the δ¹⁵N values of NO_x and HONO, and a trend for HNO₃ for the March test case, but the size of the effect was relatively small (e.g., <0.4 ‰; Fig. 11). At the start of the simulation (03:00 LT) there is no HNO₃, and therefore the initial HNO₃ is produced via OH production of HNO₃ (Reaction R39); δ¹⁵N values of HNO₃ decreased from 0.35 ‰ to 0.2 ‰ during the night. The pattern is because of increasing the importance of Reactions (R91)–(R97) in HNO₃ production at night. The smallness of the effect is because α values are all relatively small, the average δ for the NO₃+ VOC is about −4 ‰, and the amount of HNO₃ produced via these pathways is relatively small (around 2.6 % of 24 h HNO₃). The first source of the HNO₃ in the simulation (03:00 to 06:00 LT) is the NO₃ + VOC reactions and results in a slight negative δ¹⁵N in HNO₃ values (−0.01 ‰). This leaves the residual NO${}_{\mathrm{3}}^{-}$ ¹⁵N enriched that is then photolyzed into NO₂ at sunrise and used NO₂+ OH → HNO₃ production resulting in slight positive δ¹⁵N values (+0.35 ‰) (Fig. 11). The range of the diurnal HNO₃ δ¹⁵N oscillation dampens as the fraction of emitted NO that has been converted to HNO₃ has increased over time. The diurnal and multiday values in δ¹⁵N of HNO₃ did not significantly change during the winter and summer simulations (Fig. 12) run with and without the KIE for Reactions (R91)–(R97), similar to those in Fig. 11. In conclusion, although there is some δ¹⁵N effect associated with NO₃+ VOC chemistry, it is much smaller than the effects associated with the Leighton cycle, NO₂+ OH, and NO_x equilibrium.

Figure 12 The difference in δ¹⁵N(HNO₃) values when NO₃+ VOC → HNO₃ reactions are included and excluded, for 1 March simulation, relative to 1 June simulation and 1 Jan simulation. The 5 d simulation was under the conditions list in Table S3e.

3.2.2 The δ¹⁵N values of NO_x, HONO, and HNO₃ due to N₂O₅ reactions

The effect on the δ¹⁵N values of NO_x, HNO₃, and HONO associated with the EIE of N₂O₅ heterogeneous hydrolysis was also tested. 1 March simulations with N emissions and $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{0.1}$ s⁻¹ were run that included the isotope effects of the Leighton cycle (Reactions R1 and R48), NO_x isotope exchange (Reaction R238), OH production of HNO₃ (Reaction R39), and the N₂O₅ EIE (Reactions R53–R54) KIE (Reaction R239) (Table S5), as well as NO emissions. These simulations were compared to an identical simulation but where the ${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$ was set to be equal to 1.0. This ensured that the NO_y chemistry was not altered when comparing the two simulations (i.e., ${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.029}$ vs. ${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.0}$). The effect of N₂O₅ chemistry on the δ¹⁵N values of NO₂ and HNO₃ was investigated. Similar to the 1 March NO₃+ VOC tests, simulations with Reactions (R1), (R39), (R48), (R238), and (R239) isotope effects active were run and then compared to simulations with the same conditions but with Reaction (R239) turned off. In addition, March simulations were run using three different $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$ values (0.01, 0.1, and 1) and compared to each other in order to test the range of NO₂ and HNO₃ δ¹⁵N values that could be generated solely by heterogeneous N₂O₅ hydrolysis.

The average daily δ¹⁵N values of HNO₃ exhibit some diurnal oscillations that roughly reach a steady-state average value after simulation day 2. At that point HNO₃ has a δ¹⁵N = +2.5 ‰ relative to the ${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.0}$ simulation. In contrast the NO₂ δ¹⁵N values oscillate diurnally by about ±2 ‰ around an average daily difference of about −8 ‰. This change is due to the Reactions (R53)–(R54) equilibrium, which predicts ¹⁵N enrichment in N₂O₅ (and thus HNO₃) and depletion in NO₃ and NO₂. The N₂O₅ produces HNO₃ with the highest δ¹⁵N difference ($\sim +\mathrm{29}$ ‰) during the first simulation morning. This is because all of the initial HNO₃ is produced by N₂O₅ due to the 03:00 LT simulation start time. The roughly steady-state HNO₃ δ¹⁵N value of +2.5 ‰ is a consequence of the fact that when ${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.0}$ HNO₃ is being produced by N₂O₅ at 0 ‰ and when ${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.029}$ it is being produced at +29 ‰. The ratio of this simulated +2.5 ‰ value and N₂O₅ enrichment factor of +29 ‰ yields 0.086, the fraction of HNO₃ produced by N₂O₅. This is similar to the fraction of HNO₃ produced in simulations when the N₂O₅ reaction was active and where it is inactive, which yielded a fraction of 0.064. The difference in these fractions is because deactivating N₂O₅ chemistry changes overall NO_y chemistry and HNO₃ production (Dentener and Crutzen, 1993).

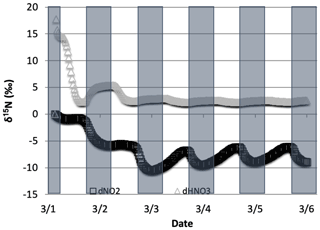
Figure 13 The difference in δ¹⁵N values of NO₂ and HNO₃ when the isotopic effect during N₂O₅ heterogeneous reactions (Reactions R53–R54, R239) is included (${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.029}$) and when it is excluded (${\mathit{\alpha }}_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.0}$). The 5 d simulation was under the conditions list in Table S3e.

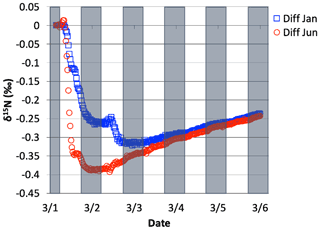
The effect of N₂O₅ chemistry on the δ¹⁵N values of NO₂ is more dynamic than HNO₃ (Fig. 13). This is mainly due to the fact that HNO₃ is continually building up over time and thus its δ¹⁵N is less susceptible to change by small additions. The oscillation in the NO₂ δ¹⁵N value becomes more negative at night, which corresponds to the increase in the HNO₃ δ¹⁵N values. This is a reflection of ¹⁵N preferentially incorporating into N₂O₅ resulting in NO₂ depleted in ¹⁵N. Similar oscillations are found in NO and HONO (data not shown) as they are connected to NO₂ buildup and decay diurnally. This suggests that nighttime partitioning of NO_y will have a small but measurable influence on daytime NO_y δ¹⁵N values. The effect of using different $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$ values had a small but measurable effect on the NO₂ and HNO₃ δ¹⁵N values. Simulations that used a $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.0}$ resulted in HNO₃ δ¹⁵N values that were about 2 ‰ lower than those run at $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{0.01}$ and 1 ‰ heavier than when $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{1.0}$ (Fig. 14). This makes sense because the mean EIE for N₂O₅ (29 ‰) is lower than that for NO₂+ OH (40 ‰); therefore as N₂O₅ produces more HNO₃ its δ¹⁵N value would decrease with respect to that of daytime HNO₃ production. Thus, the model predicts lower HNO₃ δ¹⁵N values in cold, dark polluted regions (relative to the tropics) where N₂O₅ heterogeneous hydrolysis may be the main HNO₃ production pathway (Dentener and Crutzen, 1990).

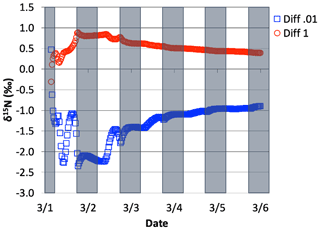
Figure 14 The difference in δ¹⁵N(HNO₃) values when the isotopic effect during N₂O₅ heterogeneous reactions is included and when it is excluded, for the simulation of $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}=\mathrm{0.1}$, relative to 0.01 and 1.0. The 5 d simulation was under the conditions list in Table S3e.

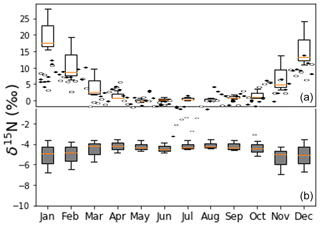
Figure 15 Panel (a) shows the observed NO${}_{\mathrm{3}}^{-}$ δ¹⁵N values of PM in the city of Tucson (Riha, 2013) and box and whisker plots values predicted by i_NRACM (1-week simulation, red line: median, box: first and third quantile, whisker is minimum and maximum). Panel (b) shows whisker plots for i_NRACM simulations with Reaction (R39) ¹⁵α=0.9971 from Freyer (1991).

3.3 Assessing i_NRACM's ability to predict in particulate NO${}_{\mathrm{3}}^{-}$

There are a number of challenges when trying to compare the i_NRACM model predictions of NO_y δ¹⁵N values with observations in real world. First, there has yet to be a study where the δ¹⁵N values of NO, NO₂, and NO${}_{\mathrm{3}}^{-}$ have been simultaneously measured. The most abundant data are on the δ¹⁵N value of NO${}_{\mathrm{3}}^{-}$ in aerosols or rainwater. Even with these studies, a direct comparison is difficult because the δ¹⁵N value of the source NO_x may be variable in space and time. The δ¹⁵N value of NO_x sources can range from −40 ‰ to +20 ‰, and both NO_x sources and NO${}_{\mathrm{3}}^{-}$ deposition will be a strong function of the transport history of the air mass that is sampled. Without a 3-D chemical transport model that includes the i_NRACM mechanism, a direct comparison with most NO${}_{\mathrm{3}}^{-}$ δ¹⁵N studies would be tenuous. In addition, most NO_y δ¹⁵N studies provide neither trace gas concentrations (NO_x, O₃, CO, VOC) nor local trace gas emissions that would be required to constrain i_NRACM for it to make an accurate prediction of secondary pollutants or δ¹⁵N values.

The most complete data set for which to evaluate the i_NRACM mechanism is from Riha (2013) in a study in Tucson, AZ, USA. In that study PM_2.5 and PM₁₀ were collected weekly (24 h period) for 1 year (2006), and the δ¹⁵N value of water soluble NO${}_{\mathrm{3}}^{-}$ from was determined (Fig. 15). It contains PM mass and NO${}_{\mathrm{3}}^{-}$ δ¹⁵N and concentration data, local measurements of the main trace gases (except VOCs) and meteorology (temperature, relative humidity, wind) were available. In addition, detailed local primary pollutant emission inventories have been developed (Diem and Comrie, 2001). Tucson is a city with little industry or power generation, so roughly 80 % of the NO_x is due to vehicles and the relative proportion of all NO_x sources is invariant throughout the year (Fig. S1). Further, Tucson is surrounded by a desert landscape and by and large not influenced by regional pollution sources outside the city. i_NRACM was initialized with observed trace gas concentrations, and NO_x and VOC emissions were based on previous work (Riha, 2013). The source NO_x δ¹⁵N value was set to −3 ‰, typical of vehicle emissions (Walters et al., 2015a) and run for 1 week from the first day of each month. The aerosol surface area used to calculate $k_{{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}}$ was based on monthly average PM mass (Fig. S2).

The predicted NO${}_{\mathrm{3}}^{-}$ (as HNO₃) δ¹⁵N values (after 36±12 h) matched remarkably well with the observed δ¹⁵N values in PM_2.5 and PM₁₀ (Fig. 15). Observed maximums were in the winter months, peaking in January at 15 ‰, close to the model maximum in January of 17 ‰. The minimum δ¹⁵N values (−2 ‰) are measured in July, similar to model predictions of 0 ‰ during July. The model captures the seasonal trend quite well, including the spring plateau. This suggests that at this location, the observed seasonal variation in PM NO${}_{\mathrm{3}}^{-}$ δ¹⁵N values can be explained by isotope effects associated with the photochemical conversion of NO_x into HNO₃. The wider range in HNO₃ δ¹⁵N values in the winter months relative to summer months is due to the difference in sunlight and oxidant loads. In winter sunlight hours are at a minimum (8.5 h versus 12.8 in June), and ozone mixing ratios are a factor of 4–5 lower compared to the summer months (Riha, 2013). This results in rapid conversion of NO_x into HNO₃ in the summer, and the ${\mathrm{HNO}}_{\mathrm{3}}/({\mathrm{HNO}}_{\mathrm{3}}+{\mathrm{NO}}_x)$ exceeds 0.90 within 2 d whereas it requires 6 d during the winter (Fig. S3). The result is that in the summer HNO₃ δ¹⁵N values rapidly approach the δ¹⁵N of the NO_x source, whereas in the winter there is greater diurnal and daily variability until the very end of the simulation. The rapid swings in HNO₃ δ¹⁵N values are thus a function of the chemical lifetime of NO_x and physical lifetime of HNO₃ with respect to wet (and dry) deposition. Thus, when the atmosphere is cleansed by regional rainfall, the isotope effects associated with photochemical oxidation will have a greater influence relative to NO_x sources, and this is a plausible explanation of rapid changes in the δ¹⁵N of rain nitrate over the course of a storm (Rose et al., 2019). Analysis of hourly HNO₃ production revealed that ∼80 % of HNO₃ is produced in the daytime, mainly by the NO₂+ OH reaction, and 20 % is produced during the night (N₂O₅ heterogenous hydrolysis). The model reproduces O₃ and NO_x concentrations rather accurately (Fig. S2), but HNO₃ concentrations are about 10 times the PM NO${}_{\mathrm{3}}^{-}$ concentration. This is not surprising because the 0-D models do not account for HNO₃ deposition, its dilution as it mixes into to the top of the boundary layer, or partitioning between aerosol and the gas phase. Indeed, seasonal differences in boundary layer height alone can dilute by a factor of 5 or higher (Riha, 2013).

4 Conclusion

We have developed the first 0-D photochemical box model for ¹⁵N compounds in the tropospheric NO_x–NO_y cycle. It was shown that of the hundreds of N reactions in the RACM mechanism only a handful significantly impact the δ¹⁵N of the main NO_y compounds (NO_x, HONO, HNO₃). Primarily these reactions are Leighton cycle reactions, NO₂ + OH, and NO_x isotope exchange, with N₂O₅ and nitrate radical reactions having a significant but minor influence on NO_y δ¹⁵N values. The model accuracy and its validation could be improved with additional research. The i_NRACM model could be refined by additional theoretical and/or experimental determination of the isotope fractionation factors for the N reactions. First and foremost, the fractionation factor for the NO₂+ OH reaction needs to be evaluated in a more robust manner. Likewise, the fractionation factor for the NO + OH, another three-body reaction, will have a large influence on HONO δ¹⁵N values, and determining its value will be key for interesting future HONO δ¹⁵N data. The fractionation factor for NO₂ photolysis requires attention given the limitation of the ΔZPE PHIFE model (Blake et al., 2003; Liang et al., 2004; Miller and Yung, 2000). On the validation end, the simultaneous measurement of δ¹⁵N in multiple NO_y compounds would expose the accuracy or limitations of the i_NRACM model in a quantitative way. The i_NRACM model reproduced observed δ¹⁵N data from a year-long study on the isotopic composition of particulate nitrate collected in Tucson, AZ. This suggests that the model, which is publicly available, could be used as an analytical tool for researchers using ¹⁵N to gain insight into NO_x sources and transformation chemistry.

The i_NRACM model is the first step in our development of a N-isotope-enabled 3-D chemical transport model (i_NCMAQ). That model will couple i_NRACM with a ¹⁵NO_x emissions model (i_NNEI) and WRF-generated transport. The i_NRACM results show that photochemistry is an important control on the δ¹⁵N of the NO_y compounds, in particular NO${}_{\mathrm{3}}^{-}$, for which there is a large and growing data set that can be used to validate the model. This is important because this suggests that δ¹⁵N in NO_y compounds could be used as a validation of different photochemical mechanisms. Further, if the photochemical effect can be deconvoluted from the observations then observed NO_y δ¹⁵N could be used as a constraint and validation of NO_x emissions inventories. Expanded to the global scale, such a model could potentially be used to investigate the cause of δ¹⁵N versions in NO${}_{\mathrm{3}}^{-}$ found in Antarctic and Greenland ice cores (Hastings et al., 2009) and linked to historical changes in NO_x emission and NO_y chemistry.