Incorporating Oxygen Isotopes of Oxidized Reactive Nitrogen in the Regional Atmospheric Chemistry Mechanism, version 2 (ICOIN-RACM2)

The oxygen isotope anomaly (Δ¹⁷O = δ¹⁷O − 0.52 × δ¹⁸O > 0) has proven to be a robust tool for probing photochemical cycling and atmospheric formation pathways of oxidized reactive nitrogen (NO_y). Several studies have developed modeling techniques to implicitly model Δ¹⁷O of NO_y molecules based on numerous assumptions that may not always be valid. Thus, these models may be oversimplified and limit our ability to compare model Δ¹⁷O values of NO_y with observations. In this work, we introduce a novel method for explicitly tracking Δ¹⁷O transfer and propagation into NO_y and odd oxygen (O_x), integrated into the Regional Atmospheric Chemistry Mechanism, version 2 (RACM2). Termed ICOIN-RACM2 (InCorporating Oxygen Isotopes of NO_y in RACM2), this new model includes the addition of 55 new species and 729 replicate reactions to represent the propagation of Δ¹⁷O derived from O₃ into NO_y and O_x. Employing this mechanism within a box model, we simulate Δ¹⁷O for various NO_y and O_x molecules for chamber experiments with varying initial nitrogen oxides (NO_x = NO + NO₂) and α-pinene conditions, revealing response shifts in Δ¹⁷O linked to distinct oxidant conditions. Furthermore, diel cycles are simulated under two summertime scenarios, representative of an urban and rural site, revealing pronounced Δ¹⁷O diurnal patterns for several NO_y components and substantial Δ¹⁷O differences associated with pollution levels (urban vs. rural). Overall, the proposed mechanism offers the potential to assess NO_y oxidation chemistry in chamber studies and air quality campaigns through Δ¹⁷O model comparisons against observations. The integration of this mechanism into a 3-D atmospheric chemistry transport model is expected to notably enhance our capacity to model and anticipate Δ¹⁷O across landscapes, consequently refining model representations of atmospheric chemistry and tropospheric oxidation capacity.

1 Introduction

Nitrogen oxides (NO_x = NO + NO₂) are essential trace gases primarily released through human activities, carrying significant implications for air quality, nutrient deposition, and the climate system (Galloway et al., 2004; Pinder et al., 2012). NO_x directly modulates atmospheric oxidation processes, consequently impacting the concentrations of various trace gases, including greenhouse gases (Prinn, 2003). Ultimately, NO_x is removed from the atmosphere as atmospheric nitrate. This global process is dominated by the formation of inorganic nitrate, encompassing nitric acid (HNO₃) and particulate nitrate (pNO₃) (Alexander et al., 2020), although the generation of organic nitrates (RONO₂) might be significant in remote and rural areas (Browne and Cohen, 2012). However, both pNO₃ and RONO₂ may not be a terminal sink for NO_x due to the potential for renoxification from photolysis (Wang et al., 2023; Gen et al., 2022). Uncertainties surrounding the rate of NO_x oxidation to atmospheric nitrate constitute a substantial source of ambiguity in models, influencing ozone (O₃) and hydroxyl radical (OH) formation, with important implications for greenhouse gas removal rates (Newsome and Evans, 2017).

The oxygen mass-independent fractionation leading to an oxygen isotope anomaly (Δ¹⁷O = δ¹⁷O − 0.52 × δ¹⁸O > 0) has emerged as a potent tool for evaluating the photochemical cycling and oxidation chemistry of NO_x and its oxidized products (NO_y = NO_x + HNO₃ + RONO₂ + nitrous acid (HONO) + peroxyacetyl nitrate (PAN) + etc.) (Alexander et al., 2020, 2009; Hastings et al., 2003; Michalski et al., 2003; Morin et al., 2011; Walters et al., 2019). While several atmospheric reactions can induce oxygen mass-independent fractionation (Röckmann et al., 1998; Velivetskaya et al., 2016), O₃ is the overwhelming source of mass-independent fractionation in the lower atmosphere, which derives from unconventional isotope effects during its formation (Gao and Marcus, 2001). In this work, we focus on the propagation of the oxygen isotope anomaly from O₃ mass-independent fractionation into NO_y and O_x molecules for applications to the lower atmosphere. The Δ¹⁷O(O₃) has been measured to be between 20 ‰ and 46 ‰ (Krankowsky et al., 2000; Mauersberger et al., 2001). This range of values has been shown to track with the pressure and temperature associated with O₃ formation (Thiemens and Jackson, 1990; Morton et al., 1990). For typical tropospheric conditions, O₃ exhibits a Δ¹⁷O between 20 ‰ and 30 ‰ (Johnston and Thiemens, 1997), with recent near-surface observations suggesting a mean Δ¹⁷O(O₃) near 26 ‰ (Vicars and Savarino, 2014; Vicars et al., 2012; Ishino et al., 2017). O₃ is also isotopically asymmetrical such that the Δ¹⁷O of its terminal and central O atoms are different (Janssen, 2005; Marcus, 2008). This intramolecular Δ¹⁷O distribution is significant because the terminal O atom of O₃ (defined as ${\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}$) is preferentially transferred during oxidation reactions involving O₃ (Bhattacharya et al., 2008; Liu et al., 2001; Michalski and Bhattacharya, 2009; Walters and Michalski, 2016). The relationship between Δ¹⁷O(O₃) and Δ¹⁷O(${\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}$) is complex, though experimental data has suggested the following relationship:

$$\begin{array}{}\text{(1)}& {\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)=\mathrm{1.5}\times {\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}\right).\end{array}$$

Applying this relationship to the assumed tropospheric mean Δ¹⁷O(O₃) of 26 ‰ would imply a ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ of 39 ‰, which is near the average of recent near-surface ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ observations of 39.3 ± 2 ‰ (Vicars and Savarino, 2014). It is important to note that there could be seasonal differences in ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ as inferred from Δ¹⁷O measurements of nitrate at Dome C (Savarino et al., 2016). On the other hand, direct observations of ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ have reported insignificant seasonal variability at Dumont d'Urville (Ishino et al., 2017). Stratospheric intrusion events could introduce O₃ with an elevated ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ due to higher stratosphere values relative to the troposphere (Krankowsky et al., 2007). Nevertheless, a recent modeling study of Δ¹⁷O of atmospheric nitrate indicated that an assumed ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value of 39 ‰, reasonably reproduced global tropospheric observations (Alexander et al., 2020). Further, recent chamber simulations have reported a Δ¹⁷O(NO₂) that reached as high as 41.2 ‰ (Blum et al., 2023), which is within the measurement uncertainty of the assumed ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value of 39.3 ± 2 ‰, assuming NO₂ formation to be dominated by NO reaction with O₃. Thus, while there may be some unresolved uncertainty regarding the ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value, an assumed tropospheric average of 39.3 ± 2 ‰ should reasonably approximate Δ¹⁷O propagation into NO_y molecules in the lower troposphere. In contrast, most other oxygen-bearing atmospheric molecules, such as oxygen (O₂), water (H₂O), and peroxy radicals (RO₂ or HO₂), possess (or are expected to possess) Δ¹⁷O values near 0 ‰ (Lyons, 2001). These large Δ¹⁷O differences enable the quantitative tracking of the influence of O₃ in NO_x oxidation chemistry.

Past observations of Δ¹⁷O in atmospheric nitrate, which includes HNO₃, pNO₃, and wet-deposited nitrate (${{\mathrm{NO}}_{\mathrm{3}}^{-}}_{\left(\mathrm{aq}\right)}$), have generally shown marked seasonal variations, reflecting shifts between O₃ and HO_x chemical regimes influencing NO_x photochemical cycling and atmospheric nitrate production (Kim et al., 2023; Michalski et al., 2012, 2003). However, harnessing the full diagnostic potential of Δ¹⁷O observations necessitates a model framework that can accurately assess and refine the representation of nitrate chemistry while linking it to nitrogen deposition and air quality. Several 0-D box models and a single 3-D global atmospheric chemistry model have been developed to simulate Δ¹⁷O (Michalski et al., 2003; Morin et al., 2011; Alexander et al., 2020, 2009). These models often rely on implicit tagging of NO₂ and HNO₃ production rates, underpinned by assumptions regarding oxygen isotope mass balance calculations, NO_x photochemical cycling dynamics, and Δ¹⁷O values of reactive oxygen species (O_x).

Table 1 Summary of the major formation pathways of several NO_y components, reaction types, and their expected Δ¹⁷O values based on oxygen isotope mass balance. X refers to halogens (Br, Cl, and I), and HC refers to hydrocarbons.

^∗ Δ¹⁷O calculated from the nitrooxy (-NO₃) functional group.

While most existing Δ¹⁷O measurements pertain to atmospheric nitrate from deposition and filter samples, our capability to measure Δ¹⁷O in other NO_y molecules has been rapidly expanding (Albertin et al., 2021; Blum et al., 2023). Based on oxygen isotope mass balance principles, substantial Δ¹⁷O variations are anticipated among different NO_y molecules, including NO₂, HONO, peroxy nitrates (RO₂NO₂), organic nitrates (RONO₂), and HNO₃, contingent upon their formation pathways (Table 1). These mass balance considerations necessitate precise knowledge of the Δ¹⁷O values for several NO_y and O_x molecules. Conventional model approaches have assumed that Δ¹⁷O of OH, RO₂, and HO₂ are approximately equal to 0 ‰ due to water vapor isotope exchange or transfer of O atoms from atmospheric O₂ (Michalski et al., 2012; Barkan and Luz, 2003). However, some of these assumptions are not valid for all relevant atmospheric conditions, such as under low relative humidity and high NO_x conditions, in which the chemical reactivity of OH could be higher than its chemical lifetime to achieve isotope equilibrium with H₂O (Michalski et al., 2012). Further, Δ¹⁷O(NO) is commonly assumed to be equal to Δ¹⁷O(NO₂) due to its rapid photochemical cycling, such that the Δ¹⁷O values of NO and NO₂ reflect the relative contributions of the oxidants involved in NO_x photochemical cycling (Alexander et al., 2020, 2009; Michalski et al., 2003; Morin et al., 2011). However, recent diel observations of δ¹⁸O(NO₂) (which tracks with Δ¹⁷O) and Δ¹⁷O(NO₂) reveal that this assumption is not universally valid due to substantial nocturnal NO emissions close to the surface (Walters et al., 2018; Albertin et al., 2021). The freshly emitted NO, with a presumed Δ¹⁷O of 0 ‰, would dilute the residual Δ¹⁷O of NO_x from the daytime. The nocturnal primary emissions of NO_y components, including NO, NO₂, and HONO significantly impacts our ability to model Δ¹⁷O using implicit methods in polluted regions, employing prior modeling techniques and oxygen isotope mass balance calculations. This modeling limitation presently impedes our capacity to leverage models for comparison with Δ¹⁷O observational constraints quantitatively to improve understanding of regional and global NO_x oxidation chemistry.

This study is dedicated to addressing uncertainties in modeling Δ¹⁷O for various NO_y molecules. We introduce a novel gas-phase chemical mechanism, designated “InCorporating Oxygen Isotopes of NO_y in RACM2”, built upon the foundation of the Regional Atmospheric Chemistry Model, version 2 (RACM2) (Goliff et al., 2013). This innovative mechanism explicitly traces the transfer and propagation of Δ¹⁷O from O₃ into NO_y molecules, with important future implications for chamber experiments and air quality studies.

2 Methods

2.1 ICOIN-RACM2 description

The Incorporating Oxygen Isotopes of Oxidized Reactive Nitrogen in the Regional Atmospheric Chemistry Mechanism, version 2 (ICOIN-RACM2), was based on the widely used RACM2 gas-phase chemical mechanism framework (Goliff et al., 2013). The RACM2 mechanism was developed to be able to simulate remote to polluted conditions from the surface to the upper troposphere. The mechanism includes 46 reactions to represent inorganic chemistry. The mechanism aggregates organic reactions based on the magnitude of emission rates, similarities in functional groups, and the compounds' reactivity (Stockwell et al., 1997) and consists of 54 stable organic species, 42 organic intermediates, 317 reactions, including 24 photolysis reactions. Overall, the RACM2 simulated concentrations of gas-phase products compare favorably to environmental chamber data (Goliff et al., 2013).

Table 2 Summary of the considered O exchange reactions and reaction rates in the ICOIN-RACM2 mechanism. These reactions were adapted from Lyons (2001).

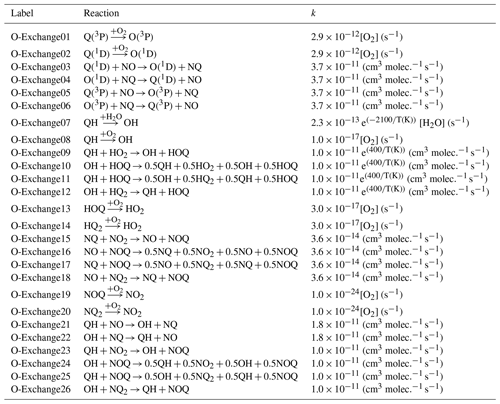
To simulate Δ¹⁷O in various NO_y and O_x molecules, the transfer and propagation of the oxygen isotope anomaly deriving from O₃ were explicitly modeled in the employed chemical mechanism. Previously, a study developed a kinetic model that explicitly tracks the ¹⁶O, ¹⁷O, and ¹⁸O abundance involving NO_x/O₃/O₂ reactions (Michalski et al., 2014). Here, we have adapted and simplified this model framework to explicitly track the transfer and propagation of O atoms derived from the terminal end of O₃ without simulating and tracking the absolute ¹⁶O, ¹⁷O, and ¹⁸O abundances, which can be tedious to employ in a detailed chemical mechanism. Our approach tagged O atoms transferred from O₃ as “Q” and tracked the interactions and propagation of “Q” among NO_y and O_x isotopologues using mass balance and considering isotopologue reaction stoichiometry. The tagging of O isotopologues was not conducted for large O reservoirs, including O₂ and H₂O. The reaction mechanism involved reactions with a single tagged O isotopologue, in which one tagged O isotopologue compound was found in the reactant and product, for example, NO + O₃ → NOQ + O₂ and NQ + O₃ → NQ₂ + O₂. Additionally, the mechanism involved reactions containing multiple tagged O compounds in the reactants and products, for example, NQ + NO₃ → NOQ + NO₂. For these, multiple O-tagged isotopologue reactions, statistical probabilities, and mass balance were considered in the product distributions. The explicit tracking and propagation of “Q” in the ICOIN-RACM2 lead to the renaming of 19 reactions, the addition of 729 reactions replicated for the considered O isotopologues, and the addition of 55 oxygen isotopologues of NO_y and O_x relative to RACM2. Additionally, 26 oxygen isotope exchange reactions were added to the ICOIN-RACM2 chemical mechanism (Lyons, 2001) (Table 2).

Based on the model output of the concentrations of the oxygen isotopologues, the Δ¹⁷O of various NO_y and HO_x molecules were calculated as follows (Eq. 2):

$$\begin{array}{}\text{(2)}& {\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left(X\right)=f\left(Q\right)\times {\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right),\end{array}$$

where X refers to the various NO_y and O_x molecules and f(Q) is the fractional amount of O atoms deriving from O₃ for a particular molecule (i.e., the fractional amount of “Q” atoms). The Δ¹⁷O(${\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}$) represents the Δ¹⁷O value of the terminal and transferable O atom of O₃. For the demonstration of the developed mechanism for applications to chamber simulations and tropospheric chemistry, we have utilized a constant ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value of 39.3 ± 2 ‰ based on near-surface-level collections of O₃ on a nitrite coated filter (Vicars and Savarino, 2014; Ishino et al., 2017). This ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value was recently utilized in the global modeling of Δ¹⁷O of atmospheric nitrate, demonstrating reasonable agreement between model simulation and observations of tropospheric nitrate (Alexander et al., 2020). The ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ could have temporal variability and be influenced by stratospheric intrusion events, which could introduce O₃ with a higher ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value. The developed model framework is highly flexible, and the user may apply a different ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ than chosen for our model simulations, which will allow users to investigate both the chemical and ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ variability on Δ¹⁷O of NO_y and O_x species when interpreting field observations. The f(Q) for the various considered molecules is calculated as follows (Eq. 3):

$$\begin{array}{}\text{(3)}& f(Q,X)={\displaystyle \frac{{\sum }_{i=\mathrm{1}}^{j}i\cdot \left[Z_{\text{ with }iQ}\right(X\left)\right]}{{\sum }_{i=\mathrm{0}}^{j}j\cdot \left[Z\right(X\left)\right]}},\end{array}$$

where Z represents the oxygen isotopologues of molecule X, which can contain or not contain Q (Z_with iQ); i represents the number of Q isotopes present in each Z of X; and j represents the maximum number of Q isotopes that can exist in X. Overall, this equation considers the distribution of Q isotopes within different arrangements and calculates the fraction of Q isotopes in the molecule relative to the total number of oxygen atoms. While our mechanism and application is focused on evaluating the propagation of oxygen isotope mass-independent fractionation from O₃ into NO_y and O_x, the model could be adapted for tracking other potential oxygen mass-independent fractionation, such as HO₂ + HO₂ or CO + OH reactions (Röckmann et al., 1998; Velivetskaya et al., 2016), by adjusting the product distribution of “Q” and “O”, such that the fraction of “Q” once scaled by the chosen ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value would match the intended Δ¹⁷O value associated with the oxygen mass-independent fractionation. Previous experiments have reported an increase in Δ¹⁷O(H₂O₂) as the initial O₂ concentrations increased (Velivetskaya et al., 2016). This result was concluded to reflect the increased role of O₃ reactions in H₂O₂ formation, which is already tracked in our mechanism. The CO + OH reaction, producing a Δ¹⁷O in the residual CO, would be extremely unlikely to affect the Δ¹⁷O of NO_y or O_x due to the long atmospheric lifetime of CO relative to NO_y or O_x. Therefore, we did not explicitly test these reactions' influence on Δ¹⁷O of NO_y or O_x in this work but could easily be adapted in future iterations of the model.

The RACM2 mechanism is a gas-phase mechanism and does not include heterogeneous reactions, which could limit the ICOIN-RACM2 mechanism's ability to accurately simulate Δ¹⁷O values, particularly of HONO and HNO₃ (Table 1). Gas-phase mechanisms are often used in larger chemical transport models that also include aerosol modules to calculate heterogeneous chemistry reaction rates. When utilizing ICOIN-RACM2 to simulate Δ¹⁷O values (and RACM2 for simulating concentrations) in box models that lack aerosol modules, appropriate reactions should be included using pseudo-first-order reaction rate constants to calculate heterogeneous hydrolysis. However, estimating the heterogeneous reaction rates is not trivial and depends on the molecular speed; uptake coefficients, which depend on aerosol chemical composition; and surface area density. These reaction rates may need to be treated in a case-by-case circumstance. Since the ICOIN-RACM2 mechanism does not model particulate nitrate, we cannot model its photolysis, which could limit our ability to simulate Δ¹⁷O(HONO). Additionally, our gas-phase mechanism does not include NO₂ heterogeneous reactions, which could also be an important source of HONO (Chai et al., 2021). Users interested in accurately simulating Δ¹⁷O(HONO) may need to consider adding relevant reactions. Still, a future comparison between Δ¹⁷O(HONO) observations and model simulations based on the ICOIN-RACM2 framework should provide pivotal insight into HONO formation.

Table 3 Summary of the precursor concentrations for the box model simulations of α-pinene and NO photochemical oxidation chamber experiments. All experiments were simulated at a fixed temperature and relative humidity of 22 °C and 1 %, respectively.

2.2 Box model description

The ICOIN-RACM2 mechanism was utilized in the Framework for 0-D Atmospheric Modeling (F0AM) box model (Wolfe et al., 2016). This box model presents a high degree of flexibility, allowing it to be seamlessly adapted for a wide range of simulation scenarios, and can perform online computation of photolysis frequencies. The ICOIN-RACM2 mechanism was developed for use in the F0AM. In this work, the F0AM model was utilized to illustrate the capacity of the ICOIN-RACM2 mechanism for simulating Δ¹⁷O values from photochemical chamber experiments and steady-state diel cycles.

2.2.1 Chamber simulations

Box model simulations were conducted to evaluate α-pinene and NO_x chemistry under various initial conditions that included variable [α-pinene]:[NO_x] ratios (Table 3). These simulations were conducted using similar initial volatile organic compound (VOC) and H₂O₂ levels utilized in recently conducted chamber experiments (Takeuchi and Ng, 2019). We have also varied the initial VOC and NO_x concentration levels to look at the impact of changing initial conditions and model chemistry on Δ¹⁷O values. As α-pinene is an important monoterpene, and its oxidation in the presence of NO_x constitutes an important mechanism of coupled biogenic–anthropogenic interaction, it has important consequences for air quality, climate, global reactive nitrogen budget, and secondary organic aerosols (SOAs) (Romer et al., 2016; Zare et al., 2018; Ng et al., 2017). The model was initiated for each experiment using NO, α-pinene, and H₂O₂ (OH precursor) concentrations. The α-pinene and H₂O₂ concentrations were fixed at 25 and 2000 ppb, respectively, while the initial NO concentrations were varied from 5 to 125 ppb to simulate oxidation chemistry in a range of [α-pinene]:[NO_x] conditions (Table 3). The pressure, temperature, and relative humidity were fixed at 1013 mbar, 295 K, and 1 %, respectively. The measured chamber light flux data from the Georgia Institute of Technology, Environmental Chamber Facility was also utilized.

The model was run for 4 h for each simulated experiment. Both gas and particle chamber wall loss were not considered in the chamber simulation comparison. Monoterpene organic nitrate hydrolysis can be an important loss process and formation pathway of HNO₃ (Zare et al., 2018; Fisher et al., 2016; Takeuchi and Ng, 2019; Wang et al., 2021) but was not considered in the model because of the low relative humidity conditions. Additionally, heterogeneous pathways leading to the production of HNO₃, such as N₂O₅, were not included. For the hypothetical simulations, this should not impact the reliability of the predictions due to the photochemical conditions of the simulated chamber experiments, low relative humidity, and high organic carbon content of produced particles, which would be reasonably expected to lead to a low N₂O₅ uptake coefficient (Escorcia et al., 2010). When utilizing the ICOIN-RACM2 mechanism to simulate chamber experimental Δ¹⁷O data, gas and particle wall loss, organic nitrate hydrolysis, and NO_y heterogeneous reactions should be considered, but it will depend on the chamber and reaction conditions and should be treated in a case-by-case circumstance. The model simulations evaluated the Δ¹⁷O temporal variation of NO₂, HONO, monoterpene-derived organic nitrate (ONIT), HNO₃, OH, and HO₂ and investigated their changes in response to the experimental oxidant conditions.

Table 4 Conditions for the box model simulations of the diel cycle for two summertime scenarios. The simulations were conducted at a fixed elevation of 0 km, temperature of 298 K, pressure of 1013.25 mbar, and on 21 June 2015, in Providence, RI (41.82° N, 71.41° W). The scenarios were adapted from Stockwell et al. (1997).

2.2.2 Diel variations

Box model simulations were also conducted in steady-state diel cycles for two summertime scenarios. These scenarios (Case 19 and Case 20) were based on previous case studies utilized to evaluate the RACM and RACM2 mechanism (Stockwell et al., 1997; Goliff et al., 2013). Briefly, Case 19 represents a somewhat polluted atmosphere with emissions of NO_x and organic compounds, and Case 20 represents a relatively clean atmosphere with the initial concentrations and emission rates of NO_x and organic compounds reduced by a factor of 10 (Table 4). These scenarios would be analogous to near-surface summertime environments at an urban (i.e., Case 19) and rural (i.e., Case 20) environment. The box model simulations were conducted for the initial conditions and with a fixed elevation of 0 km, temperature of 298 K, pressure of 1013.25 mbar, and for 21 June as previously described (Stockwell et al., 1997). The simulations were conducted for Providence, RI (41.82° N, 71.41° W), and the diel photolysis rates were calculated using the online module in F0AM (Wolfe et al., 2016). To avoid the buildup of concentrations in the box model, a dilution lifetime of 24 h was incorporated into the simulations, as previously described (Wolfe et al., 2016). The model simulations were run for 5 d at a 1 h interval. The first 2 d of the simulation were used as a spin-up period, and the diel cycles were evaluated based on the average of the final 3 d of the simulation.

Table 5 Summary of the NO_y heterogeneous reactions, assumed uptake coefficients, and the reference or calculated pseudo-first-order reaction rates adapted in the RACM2(het) and ICOIN-RACM2(het) chemical mechanisms.

^a Adapted from Holmes et al. (2019). ^b Calculated by scaling the k_het (N₂O₅) based on the relative γ. ^c Taken from the MCM v3.3.1 for general ambient scenarios.

Figure 1 Comparison of the RACM2 (dashed yellow line) and ICOIN-RACM2 (solid purple line) mechanisms for simulating concentrations of several molecules for (a) chamber experiment 3 and (b) diel cycle Case 19.

3 Results and discussion

3.1 Mechanism evaluation

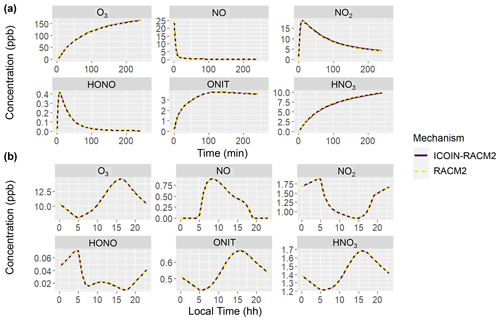
The efficacy of the isotope tagging methodology was assessed through a comparative analysis of molecule concentrations using both the RACM2 and ICOIN-RACM2 mechanisms. For molecules encompassing oxygen isotopologues explicitly considered in the ICOIN-RACM2 mechanism, the concentrations were derived by summing the isotopologue concentrations (Eq. 4).

$$\begin{array}{}\text{(4)}& \left[X\right]=\sum _i[Z{]}_i,\end{array}$$

where [X] refers to the concentration of a molecule with oxygen isotopologues, i refers to the unique oxygen isotopologues, and [Z_i] refers to the concentration of the ith isotopologue. Both the RACM2 and ICOIN-RACM2 mechanisms simulated identical concentrations across both simulated scenarios: the hypothetical chamber experiments and the diurnal variation case study during the summer period (Fig. 1). This congruence in results aligns with expectations, as the isotope tagging approach implemented in the ICOIN-RACM2 is designed not to alter the chemical kinetics governing gas-phase reactions. Indeed, by definition, the presence of isotopes should remain inert with regard to chemical reactivity. This comparative analysis serves as a robust validation of the isotope tagging methodology's ability in simulating Δ¹⁷O values while maintaining the chemical reactivity stipulated by the RACM2 mechanism.

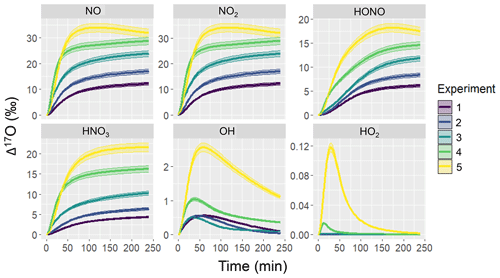
Figure 2 Simulation of Δ¹⁷O for several NO_y and O_x molecules including NO, NO₂, HONO, HNO₃, OH, and HO₂ for the various hypothetical chamber experiments (color coded). The solid line represents the modeled Δ¹⁷O value and the shaded region corresponds to the propagated uncertainty associated with the chosen ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value of 39.3 ± 2 ‰. The experimental initial conditions are provided in Table 3, which include a starting NO concentration of 5, 12.5, 25, 62.5, and 125 ppb for experiments 1, 2, 3, 4, and 5, respectively.

3.2 Chamber simulations of NO_x/α-pinene chemistry

The simulated Δ¹⁷O values derived from the hypothetical chamber simulations reveal significant temporal variations (Fig. 2). Overall, there were significant differences in Δ¹⁷O values across the considered molecules that increased in the sequence of HO₂, OH, HNO₃, HONO, and NO ≈ NO₂. In the photochemical model simulations, the Δ¹⁷O(NO) ≈ Δ¹⁷O(NO₂) due to both rapid NO_x photochemical cycling and NO/NO₂ isotope exchange. Among the considered NO_y molecules, the initial Δ¹⁷O values start at 0 ‰ and subsequently rises due to the generation of O₃ and subsequent propagation into the NO_y components, which leads to heightened Δ¹⁷O values. The extent of Δ¹⁷O elevation was determined to be contingent upon the initial chamber conditions, becoming more pronounced with increasing initial NO to α-pinene ratios for Δ¹⁷O of NO, NO₂, HONO, and HNO₃. In contrast, Δ¹⁷O(HO₂) was nearly negligible, aligning with common assumptions in other Δ(¹⁷O) models (Alexander et al., 2020, 2009; Michalski et al., 2003; Morin et al., 2011). Similarly, Δ¹⁷O(OH) generally maintained close proximity to 0 ‰, in line with typical assumptions in other Δ(¹⁷O) models (Alexander et al., 2020, 2009; Michalski et al., 2003; Morin et al., 2011); however, there were instances that deviated from this trend. Notably, higher Δ¹⁷O(OH) values were observed as the initial NO_x relative to biogenic VOC (BVOC) concentrations increased. This occurrence can be attributed to the increased significance of oxygen isotope exchange between NO₂ and OH for the higher initial NO_x experimental conditions.

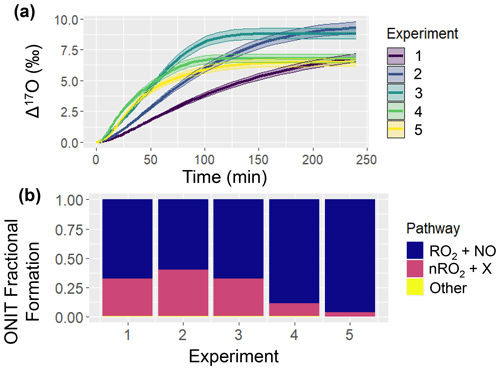
Figure 3 Simulation of ONIT chemistry for the various considered chamber experiments including (a) Δ¹⁷O of ONIT and (b) ONIT fractional formation pathways. The solid line represents the modeled Δ¹⁷O value, and the shaded region corresponds to the propagated uncertainty associated with the chosen ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value of 39.3 ± 2 ‰ (a). The experimental initial conditions are provided in Table 3, which include a starting NO concentration of 5, 12.5, 25, 62.5, and 125 ppb for experiments 1, 2, 3, 4, and 5, respectively.

Figure 4 Diel simulation of Δ¹⁷O for several NO_y and O_x molecules including NO, NO₂, HONO, HNO₃, OH, and HO₂ for the various hypothetical summertime cases (color coded). The solid line represents the modeled Δ¹⁷O value, and the shaded region corresponds to the propagated uncertainty associated with the chosen ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value of 39.3 ± 2‰. The shading corresponds to daytime (light yellow) and nighttime (dark blue) conditions.

An intriguing observation was that the simulated Δ¹⁷O(ONIT) values remained unaffected by the chamber's initial conditions (Fig. 3). This observation underscores the diverse ONIT formation pathways present in the experiments, encompassing a Δ¹⁷O(ONIT) low-end pathway involving the α-pinene peroxy radical (APIP; a type of RO₂) + NO and a high-end pathway involving nitrooxy peroxy (nRO₂) deriving from α-pinene + NO₃ reactions (Table 1). We note that even though all of the simulated experiments were conducted under photochemical conditions, the model predicted some oxidation of α-pinene with NO₃. The relative proportion of these two significant ONIT formation routes exhibited substantial variability across the various experiments (Fig. 3). Generally, a higher fractional formation of ONIT occurred through the Δ¹⁷O high-end member pathway of nRO₂ + Y (where Y = HO₂, NO, nRO₂, ACO₃, MO₂) as the initial NO to α-pinene ratios were lower. Additionally, we note that the produced Δ¹⁷O(ONIT) value is a balance between the ONIT production pathway and the Δ¹⁷O(NO) (Table 1). For the lower initial [NO_x]:[α-pinene] experiments, a lower Δ¹⁷O(NO) value was simulated. The balance between Δ¹⁷O(NO) and the pathway leading to ONIT production can explain the observation that Δ¹⁷O(ONIT) was insensitive to initial conditions.

The simulated Δ¹⁷O values from the chamber experiments highlight compelling dynamics. Primarily, there were substantial differences in Δ¹⁷O values arising from different formation pathways contributing to ONIT production. The uncertain nature of branching ratios and product yields for ONIT underscores the potential utility of comparing Δ¹⁷O(ONIT) observations with model simulations, aiding in the refinement of our understanding of ONIT yields originating from APIP + NO and API + NO₃ reaction pathways. Furthermore, a significant divergence between HNO₃ and ONIT in terms of Δ¹⁷O was observed, particularly with heightened initial NO to α-pinene concentrations. This divergence led to considerably higher simulated Δ¹⁷O(HNO₃) than Δ¹⁷O(ONIT). This difference could potentially be utilized to help constrain the contribution of ONIT hydrolysis to HNO₃ through a comparison of observed Δ¹⁷O(HNO₃) and model-based predictions. Lastly, the model simulations underscore the potential of employing the ICOIN-RACM2 model to corroborate Δ¹⁷O values for OH and HO₂ under diverse conditions (such as concentrations, chemical composition, relative humidity, and temperature), which are commonly assumed to be near 0 ‰.

3.3 Summertime diel simulations

The simulated Δ¹⁷O diel profiles indicate interesting patterns for the various considered molecules, including NO, NO₂, HONO, HNO₃, OH, and HO₂ (Fig. 4). The Δ¹⁷O of NO, NO₂, and HONO indicates a strong diurnal pattern for both considered summertime case studies. The simulated Δ¹⁷O of NO and NO₂ indicates lower values during the nighttime and higher values during the daytime. This is due to the importance of nighttime NO emissions, such that the O atoms of NO and NO₂ are not photochemically cycled. The simulated daytime profiles of Δ¹⁷O of NO and NO₂ follow similar patterns, reflecting their fast photochemical cycling. Near sunrise, Δ¹⁷O of NO and NO₂ reaches a peak due to photochemical cycling that primarily involves O₃. As photolysis continues, there is a significant enhancement of peroxy radicals (${\mathrm{RO}}_{\mathrm{2}}/{\mathrm{HO}}_{\mathrm{2}}$), which slightly dilutes the Δ¹⁷O of NO and NO₂. Near sunset, the peroxy radical concentrations decrease, and once again NO and NO₂ predominantly photochemically cycle with O₃. During the daytime, the simulated Δ¹⁷O(NO₂) ≈ Δ¹⁷O(NO) due to the rapid NO_x photochemical cycling. However, during the nighttime, Δ¹⁷O(NO₂) was greater than Δ¹⁷O(NO) due to the role of nighttime NO emissions with an assumed Δ¹⁷O(NO) = 0 ‰. While NO and NO₂ isotope exchange would lead to Δ¹⁷O(NO) = Δ¹⁷O(NO₂), its role in influencing Δ¹⁷O depends on the concentrations of NO and NO₂, as previously discussed for δ¹⁵N of NO_x (Walters et al., 2016). In the diel model simulations, nighttime NO concentrations were less than 0.1 ppb (Fig. 1) due to its titration by O₃. Under these conditions, the rate of NO/NO₂ isotope exchange was slow relative to NO oxidation or the rate of NO primary emission, leading to a low nighttime Δ¹⁷O(NO) value for the simulation conditions of low nighttime NO_x relative to O₃ concentrations.

The predicted NO₂ diurnal cycles of elevated Δ¹⁷O during the daytime and low Δ¹⁷O during the nights are generally consistent with summertime δ¹⁸O observations (which track with Δ¹⁷O) in West Lafayette, IN, US (Walters et al., 2018), and recent diel observations of Δ¹⁷O at Grenoble, FR, during the spring (Albertin et al., 2021). However, there are some slight differences in the daytime Δ¹⁷O(NO₂) observations compared to the model simulations, in which the highest Δ¹⁷O(NO₂) occurred for samples collected between 9 am–12 pm (Albertin et al., 2021). In comparison, the model indicated the highest Δ¹⁷O(NO₂) around 6 to 8 am following the return of photolysis near sunrise. The observations indicate a subsequent daytime decay of Δ¹⁷O(NO₂) (Albertin et al., 2021). The model also indicates a daytime decay in Δ¹⁷O(NO₂) following the initial maximum Δ¹⁷O(NO₂) that coincides with the onset of photolysis; however, the model expects an increase in Δ¹⁷O(NO₂) in the late afternoon due to increased ${\mathrm{O}}_{\mathrm{3}}/{\mathrm{HO}}_x$ levels from the decrease in actinic flux. We do not intend to accurately simulate the previously reported Δ¹⁷O(NO₂) values (Albertin et al., 2021). Some of the nuanced differences between the model simulation and observations of Δ¹⁷O are likely due to differences in meteorological conditions, as the model was simulated for summertime while the observations were from springtime and for a different latitude and longitude. Further, our model neglects transport and assumes a constant emission rate, which could influence the diel Δ¹⁷O(NO₂) predictions. Nevertheless, the ICOIN-RACM2 mechanism appears to capture the general diurnal trend of Δ¹⁷O(NO₂). We envision that future adaptation of the ICOIN-RACM2 mechanism into a chemical transport model would provide useful insight for constraining NO_x photochemical cycling based on a comparison to field Δ¹⁷O(NO₂) measurements.

The diurnal variation in Δ¹⁷O(HONO) exhibits an inverse pattern compared to NO and NO₂, characterized by nocturnal maxima and daytime minima. This contrast arises from distinct formation pathways operating during daytime and nighttime. For our model simulations and conditions, HONO formation predominantly occurs via NO₂ heterogeneous reactions during the night, giving rise to a high-Δ¹⁷O(HONO) end-member (Table 1). Conversely, daytime HONO production centers around the NO + OH pathway, leading to a low-Δ¹⁷O(HONO) end-member, which dilutes the Δ¹⁷O of the formed HONO relative to Δ¹⁷O of HONO and NO (Table 1). Notably, primary emissions could significantly contribute to HONO levels but were excluded from the hypothetical summertime scenarios (Stockwell et al., 1997). If primary HONO emissions were substantial, a lower Δ¹⁷O(HONO) during the night would be anticipated due to a lack of NO_y photochemical cycling, assuming primary emissions carry a Δ¹⁷O(HONO) value of 0 ‰. Additionally, we note that the model, based on a gas-phase mechanism, does not include photolysis of pNO₃, which could be an important source of HONO (Ye et al., 2016). For future interpretation of Δ¹⁷O(HONO) observations, photolysis of pNO₃ and optimized NO₂ heterogeneous reaction rates would need to be considered.

The Δ¹⁷O of HNO₃, OH, and HO₂ had little variation in their diel profiles. The Δ¹⁷O(HNO₃) tended to converge to a value dependent on the oxidant conditions for Case 19 and Case 20. There was no significant simulated Δ¹⁷O(HNO₃) diurnal variability due to the relatively longer HNO₃ lifetime in the gas-phase mechanism relative to NO, NO₂, and HONO, which rapidly undergo photochemical cycling. In the RACM2 mechanism, the significant chemical loss pathways for HNO₃ are HNO₃ + OH and HNO₃ photolysis, which are relatively slow loss pathways, essentially “locking in” the Δ¹⁷O(HNO₃) values. Thus, due to the relatively elevated HNO₃ lifetime, the simulated Δ¹⁷O(HNO₃) builds up toward a steady-state value. While the modeled diel Δ¹⁷O(HNO₃) indicated no substantial diurnal variations, several field studies have indicated significant diurnal variability of Δ¹⁷O(pNO₃) in polluted mega-cities (Zhang et al., 2022), as well as off the coast of California (Vicars et al., 2013). Commonly, Δ¹⁷O(HNO₃) is thought to be equal to Δ¹⁷O(pNO₃) due to the thermodynamic equilibrium between HNO₃ and pNO₃ in the fine aerosol mode (Alexander et al., 2009). However, recent data would suggest that Δ¹⁷O(HNO₃) may not always be equal to Δ¹⁷O(pNO₃) due to contributions of pNO₃ in the coarse aerosol phase that may not achieve thermodynamic equilibrium with HNO₃ (Kim et al., 2023). If we consider that the Δ¹⁷O(pNO₃) diurnal variability should follow Δ¹⁷O(HNO₃), the discrepancy between model and observations of diurnal variability would suggest that the lifetime of pNO₃ in these previous studies must be shorter than predicted in our model for HNO₃. Our model simulation was conducted using a gas-phase mechanism within a simple box model framework. Potentially important pNO₃ loss processes not included in our model include pNO₃ photolysis and wet or dry deposition. These processes should not alter the Δ¹⁷O of pNO₃ but could reduce the lifetime of pNO₃, leading to a significant diurnal variation in Δ¹⁷O. Additionally, our model simulation does not include transport or changes in boundary layer height and break up of the nocturnal boundary layer, which could also influence Δ¹⁷O diurnal variations of HNO₃ and pNO₃.

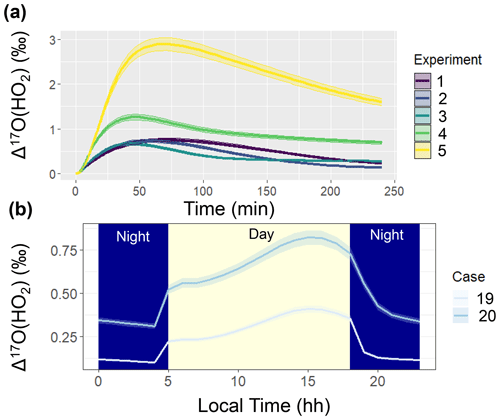
Figure 5 Simulated Δ¹⁷O(HO₂) when not considering oxygen isotope exchange between HO₂ and O₂ in the ICOIN-RACM2 mechanism (i.e., O-Exchange13 and O-Exchange14 in Table 2) for (a) the considered chamber simulations (color coded) and (b) the diel simulations for hypothetical summertime cases (color coded). The solid line represents the modeled Δ¹⁷O value, and the shaded region corresponds to the propagated uncertainty associated with the chosen ${\mathrm{\Delta }}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}^{\mathrm{term}}\right)$ value of 39.3 ± 2 ‰. The shading corresponds to daytime (light yellow) and nighttime (dark blue) conditions for the diel simulations (b).

The simulated Δ¹⁷O of OH and HO₂ was near 0 ‰ for the entire simulation. We note that the simulated Δ¹⁷O(HO₂) was lower than previous Δ¹⁷O(HO₂) simulations (Morin et al., 2011), which tended to be between 1 ‰ to 2 ‰. This difference is because we have included oxygen isotope exchange reactions involving O₂ and HO₂ (Lyons, 2001) (i.e., O-Exchange13 and O-Exchange14 in Table 2) in the ICOIN-RACM2 mechanism, which rapidly remove Δ¹⁷O > 0 ‰ in the generated HO₂. Without including this oxygen isotope exchange reaction, the ICOIN-RACM2 modeled Δ¹⁷O(HO₂) predicts a non-zero Δ¹⁷O(HO₂) that can be as high as 3 ‰ dependent on the model conditions (Fig. 5), consistent with previous model simulations (Morin et al., 2011). While the Δ¹⁷O(HO₂) is expected to have a minor impact on the Δ¹⁷O of NO_y species (Alexander et al., 2009), we should consider the importance of the role of oxygen isotope exchange between O₂ and HO₂ influencing Δ¹⁷O(HO₂), as it will be an important source of Δ¹⁷O of H₂O₂, which is propagated into atmospheric sulfate (Savarino et al., 2000).

Comparing Case 19 and Case 20 reveals coherent diel Δ¹⁷O patterns. The primary disparity between these case studies lies in the higher Δ¹⁷O values exhibited by the considered NO_y compounds in the urban setting of Case 19 compared to the rural backdrop of Case 20 (Fig. 4). The general divergence between the urban and rural simulated Δ¹⁷O stems from the interplay between O₃ and ${\mathrm{RO}}_{\mathrm{2}}/{\mathrm{HO}}_{\mathrm{2}}$. Urban conditions (i.e., Case 19) entail greater contributions from NO_x photochemical cycling with O₃ relative to the rural environment (i.e., Case 20). An important exception to the urban vs. rural trend was for Δ¹⁷O(HO₂) when O₂/HO₂ exchange reactions were not considered in the model simulation (Fig. 5). From these simulations, the rural Δ¹⁷O(HO₂) was slightly larger than the urban simulations, reflecting differences in the involvement of O₃ in HO₂ formation between these simulations. Overall, these hypothetical simulations highlight the prospect of intriguing Δ¹⁷O variations between urban and rural settings.

4 Conclusions

This study introduces a novel gas-phase mechanism, denoted as ICOIN-RACM2, which is built upon the RACM2 gas-phase chemical mechanism framework (Goliff et al., 2013). This mechanism is designed to explicitly model the Δ¹⁷O of NO_y and O_x molecules based on quantitatively tracking the incorporation and propagation of the oxygen isotope anomaly derived from O₃. Its application is demonstrated through box model simulations encompassing diverse hypothetical scenarios. These scenarios include chamber experiments focused on α-pinene and NO photochemical oxidation and the exploration of diurnal cycles in summertime chemistry.

These initial investigations serve as a fundamental step towards advancing our comprehension of NO_y oxidation chemistry and its intricate pathways. Notably, the mechanism exhibits promising capabilities in simulating Δ¹⁷O values for multiple NO_y and O_x species. This capacity holds considerable promise for refining our insights into aspects such as ONIT formation, branching ratios, and hydrolysis dynamics. Moreover, the ICOIN-RACM2 mechanism emerges as a valuable tool for various air-quality-related objectives. It could be an invaluable tool for the assessment of primary emission strengths for HONO and the probing of urban-to-rural gradients of atmospheric oxidation chemistry. In forthcoming endeavors, this newly devised model will be instrumental in direct comparisons with Δ¹⁷O observations arising from chamber experiments and investigations tied to air quality. As techniques for analyzing Δ¹⁷O in NO_y molecules continue to advance, the model's utility is poised to expand.

An envisioned next step involves integrating the model into a broader 3-D atmospheric chemistry framework. This integration is anticipated to offer vital insights for evaluating the representation of oxidation chemistry across diverse landscapes. These endeavors have far-reaching implications, notably in fine-tuning our capacity to accurately model and predict atmospheric oxidation processes, thus enhancing our overall understanding of atmospheric oxidation capacity.